Advertisements
Advertisements
प्रश्न
Find the coordinates of the point R on the line segment joining the points P(–1, 3) and Q(2, 5) such that PR = `3/5` PQ.
उत्तर
According to the question,
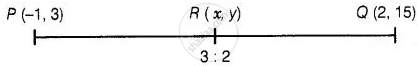
Given that,
PR = `3/5`PQ
⇒ `("PQ")/("PR") = 5/3`
⇒ `("PR" + "RQ")/("PR") = 5/3`
⇒ `1 + ("RQ")/("PR") = 5/3`
⇒ `("PQ")/("PR") = 5/3 - 1 = 2/3`
∴ RQ : PR = 2 : 3
or PR : RQ = 3 : 2
Suppose, R(x, y) be the point which divides the line segment joining the points P(–1, 3) and Q(2, 5) in the ratio 3 : 2
∴ (x, y) = `{(3(2) + 2(-1))/(3 + 2), (3(5) + 2(3))/(3 + 2)}` ...`[∵ "By internal section formula", {(m_2x_1 + m_1x_2)/(m_1 + m_2), (m_2y_1 + m_1y_2)/(m_1 + m_2)}]`
= `((6 - 2)/5, (15 + 6)/5)`
= `(4/5, 21/5)`
Hence, the required coordinates of the point R is `(4/5, 21/5)`.
APPEARS IN
संबंधित प्रश्न
Find the ratio in which the point P(x, 2) divides the line segment joining the points A(12, 5) and B(4, −3). Also, find the value of x.
Prove that the diagonals of a rectangle bisect each other and are equal.
Find the ratio in which P(4, m) divides the line segment joining the points A(2, 3) and B(6, –3). Hence find m.
The line segment joining the points M(5, 7) and N(–3, 2) is intersected by the y-axis at point L. Write down the abscissa of L. Hence, find the ratio in which L divides MN. Also, find the co-ordinates of L.
Find the length of the hypotenuse of a square whose side is 16 cm.
In what ratio is the line joining (2, -4) and (-3, 6) divided by the line y = O ?
A (30, 20) and B ( 6, -4) are two fixed points. Find the coordinates of a point Pin AB such that 2PB = AP. Also, find the coordinates of some other point Qin AB such that AB = 6 AQ.
If point P(1, 1) divide segment joining point A and point B(–1, –1) in the ratio 5 : 2, then the coordinates of A are ______
The fourth vertex D of a parallelogram ABCD, whose three vertices are A(–2, 3), B(6, 7) and C(8, 3), is ______.
A line intersects y-axis and x-axis at point P and Q, respectively. If R(2, 5) is the mid-point of line segment PQ, them find the coordinates of P and Q.
