Advertisements
Advertisements
प्रश्न
Four equal circles each of radius a, touch each other. Show that area between them is `6/7a^2`
उत्तर
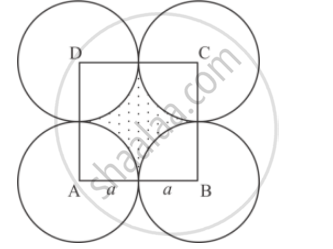
Let circles be with centres A, B, C, D]
Join A, B, C and D then ABCD is square formed with side = (a + a) = 2a
Radius = a
Area between circles = area of square – 4(area of quadrant)
(shaded region)
= (2𝑎)2 − 4 (`1/4`𝑎𝑟𝑒𝑎 𝑜𝑓 𝑐𝑖𝑟𝑐𝑙𝑒 𝑤𝑖𝑡ℎ 𝑟𝑎𝑑𝑖𝑢𝑠 ′𝑎′)
=` 4a^2 − 4 (1/4) × a^2`
= 𝑎2(4 − 𝜋)
= 𝑎2 (4 −`22/7`)
= `((28−22)/7) a^2 =6/7a^2`
∴ Area between circles =`6/7a^2.`
APPEARS IN
संबंधित प्रश्न
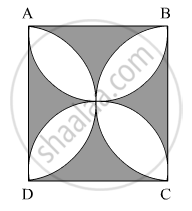
In Fig. 4, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
(use `pi=22/7`)
A wire is looped in the form of a circle of radius 28 cm. It is re-bent into a square form. Determine the length of the side of the square.
Find the area of the sector of a circle whose radius is 14 cm and angle of sector is 45º
An elastic belt is placed round the rim of a pulley of radius 5 cm. One point on the belt is pulled directly away from the centre O of the pulley until it is at P, 10 cm from O. Find the length
of the belt that is in contact with the rim of the pulley. Also, find the shaded area.
The minute hand of a circular clock is 15 cm long. How far does the tip of the minute hand move in 1 hour. (Take π = 3.14)
A plot is in the form of rectangle ABCD having semi-circle on BC. If AB = 60m and BC = 28m, find the area of plot.
The radius of a sector of a circle is 7 cm. If the measure of the arc of the sector is - three right angles; find the area of the sector in case.
The circumference of a circle is 8 cm. Find the area of the sector whose central angle is 72°.
Find the area and perimeter of the following semi-circle :
Radius= 1.4 cm
Find the diameter of the sphere for the following :
Surface Area = `576pi`cm2
