Advertisements
Advertisements
प्रश्न
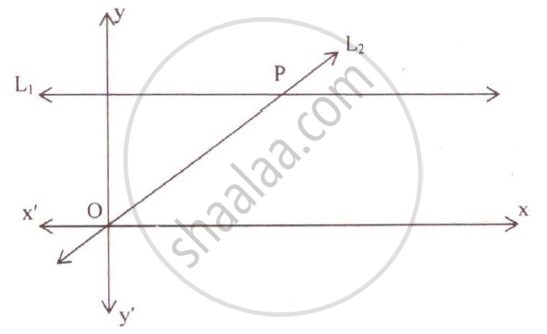
Given the equation of line L, is y = 4.
(1) Write the slope of line L2, if L2, is the bisector of angle O.
(2) Write the co–ordinates of point P.
(3) Find the equation of L2.
उत्तर
The equation of the line L1 is y = 4.
It is given that L2 is the bisector of angle O and ∠O = 90˚.
Thus, the line L2 makes an angle of 45˚ with the x-axis.
Thus, slope of line L2 = tan 45˚ = 1
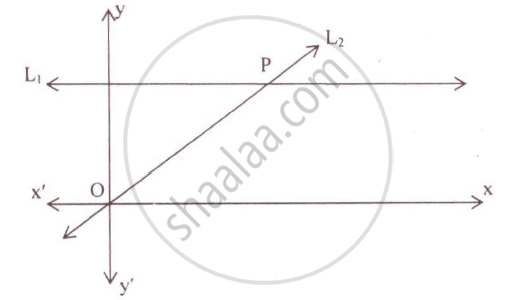
The line L2 passes through (0, 0) and its slope is 1. So, its equation is given by
y – y1 = m(x – x1)
y – 0 = 1(x – 0)
y = x
Now, the point P is the point of intersection of the lines L1 and L2.
Solving the equations y = 4 and x = y, we get x = y = 4
Thus, the coordinates of the point P are (4, 4).
APPEARS IN
संबंधित प्रश्न
Find, which of the following points lie on the line x – 2y + 5 = 0 :
(2, –1.5)
The co-ordinates of two points A and B are (-3, 4) and (2, -1) Find: the co-ordinates of the point where the line AB intersects the y-axis.
In triangle ABC, the co-ordinates of vertices A, B and C are (4, 7), (–2, 3) and (0, 1) respectively. Find the equation of median through vertex A. Also, find the equation of the line through vertex B and parallel to AC.
Find the equation of the line, whose x-intercept = −4 and y-intercept = 6
Find the equations of the line through (1, 3) and making an intercept of 5 on the y-axis.
Find the value of p if the line 3y = 5x - 7 passes through the point (p,6).
Find the equation of a line passing through (2,5) and making and angle of 30° with the positive direction of the x-axis.
Find the equation of a line passing through (2,9) and parallel to the line 3x + 4y = 11
Find the equation of a line passing through the intersection of x + 3y = 6 and 2x - 3y = 12 and parallel to the line 5x + 2y = 10
Find the value of ‘a’ for which the following points A (a, 3), B (2, 1) and C (5, a) are collinear. Hence find the equation of the line.
