Question

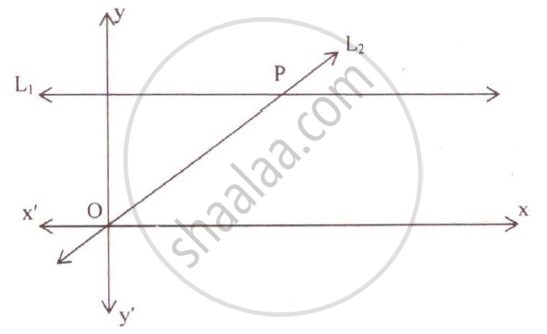
Given the equation of line L, is y = 4.
(1) Write the slope of line L₂, if L₂, is the bisector of angle O.
(2) Write the co–ordinates of point P.
(3) Find the equation of L₂.

Answer 1

The equation of the line L₁ is y = 4.
It is given that L₂ is the bisector of angle O and ∠O = 90˚.
Thus, the line L₂ makes an angle of 45˚ with the x-axis.
Thus, slope of line L₂ = tan 45˚ = 1

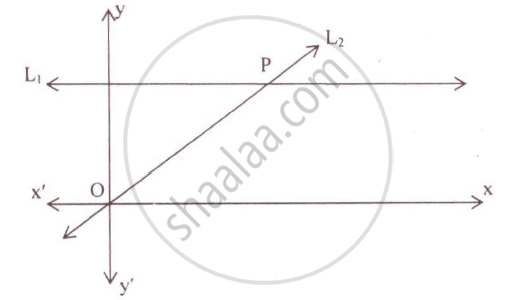
The line L₂ passes through (0, 0) and its slope is 1. So, its equation is given by
y – y₁ = m(x – x₁)
y – 0 = 1(x – 0)
y = x
Now, the point P is the point of intersection of the lines L₁ and L₂.
Solving the equations y = 4 and x = y, we get x = y = 4
Thus, the coordinates of the point P are (4, 4).