Advertisements
Advertisements
Question
A(7, −1), B(4, 1) and C(−3, 4) are the vertices of a triangle ABC. Find the equation of a line through the vertex B and the point P in AC; such that AP : CP = 2 : 3.
Solution
P divides AC in the ratio of 2 : 3
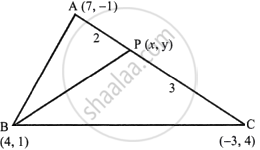
∴ Co-ordinates of P will be
`((m_1x_2 + m_2x_1)/(m_1 + m_2),(m_1y_2 + m_2y_1)/(m_1 + m_2))`
`((2(-3) + 3(7))/(2 + 3),(2 xx 4 + 3 xx (-1))/(2 + 3))`
= `((-6 + 21)/5, (8 - 3)/5)`
= `(15/5, 5/5)`
= (3, 1)
∴ Slope of line passing through B and P
= `(y_2 - y_1)/(x_2 - x_1)`
= `(1 - 1)/(3 - 4)`
= `0/(-1)`
= 0
∴ Equation of the required line is given by y – y1 = m(x – x1)
`=>` y – 1 = 0(x – 4)
`=>` y – 1 = 0
`=>` y = 1
APPEARS IN
RELATED QUESTIONS
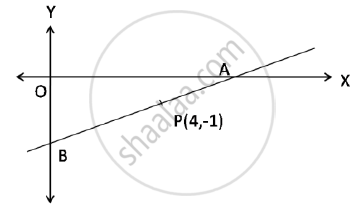
A line AB meets X – axis at A and Y –axis at B. P (4, -1) divides AB in the ratio 1 : 2.
1) Find the coordinates of A and B.
2) Find the equation of the line through P and perpendicular to AB.
Find, which of the following points lie on the line x – 2y + 5 = 0 :
(–5, 0)
State, true or false :
The line `x/ 2 + y/3 = 0` passes through the point (2, 3).
The vertices of a ΔABC are A(3, 8), B(–1, 2) and C(6, –6). Find:
(i) Slope of BC
(ii) Equation of a line perpendicular to BC and passing through A.
Find the value of m if the line 2x + 5y + 12 = 0 passes through the point
( 4,m ).
Find the value of a if the line 4 x = 11 - 3y passes through the point (a, 5)
The line segment formed by the points (3, 7) and (-7, z) is bisected by the line 3x + 4y =18. Find the value of z.
Find the inclination of a line whose gradient is 0.4663
ABCD is rhombus. The coordinates of A and C ae (3,7) and (9,15). Find the equation of BD.
A and B are two points on the x-axis and y-axis respectively.

- Write down the coordinates of A and B.
- P is a point on AB such that AP : PB = 1 : 1.
Using section formula find the coordinates of point P. - Find the equation of a line passing through P and perpendicular to AB.
