Advertisements
Advertisements
प्रश्न
If ∆MNO ≅ ∆DEF, ∠M = 60° and ∠E = 45° then find the value of ∠O
उत्तर
Given ∆MNO ≅ ∆DEF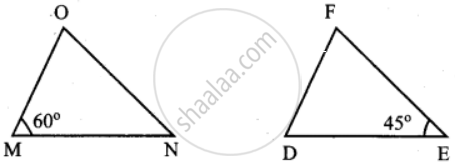
∴ Corresponding parts of the congruent triangle are congruent.
∠M = ∠D = 60° ...[given ∠M = 60°]
∠N = ∠E = 45° ...[given ∠E = 45°]
∠O = ∠F
In triangle MNO, sum of the three angle is 180°
∠M + ∠N + ∠O = 180°
60° + 45° + ∠O = 180°
105° + ∠O = 180°
∠O = 180° – 105° = 75°
Value of ∠O = 75°
APPEARS IN
संबंधित प्रश्न
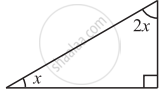
Find the value of the unknown x in the following diagram:
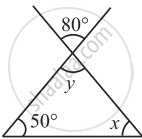
Find the value of the unknown x and y in the following diagram:
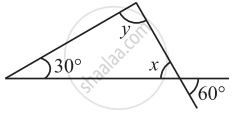
Find the value of the unknown x and y in the following diagram:
Find the value of the unknown x and y in the following diagram:

In the following triangle, find the value of x
In the following triangle, find the value of x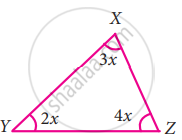
In the given figure, which of the following statement is true?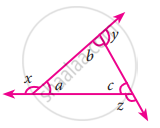
Prove that through a given point, we can draw only one perpendicular to a given line.
[Hint: Use proof by contradiction].
In the following figure, ∠Q > ∠R, PA is the bisector of ∠QPR and PM ⊥ QR. Prove that ∠APM = `1/2` (∠Q – ∠R).
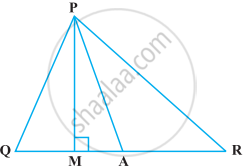
In the given figure, if ST = SU, then find the values of x and y.

