Advertisements
Advertisements
प्रश्न
In a circle of radius 17 cm, two parallel chords of lengths 30 cm and 16 cm are drawn. Find the distance between the chords,
if both the chords are:
(i) on the opposite sides of the centre;
(ii) on the same side of the centre.
उत्तर
Let O be the center of the circle and AB and CD be the two parallel chords of length 30 cm and 16 cm respectively.
Drop OE and OF perpendicular on AB and CD from the center O.

OE ⊥ AB and OF ⊥ CD.
∴ OE bisects AB and OF bisects CD. ...( Perpendicular is drawn from the centre of a circle to a chord bisects it. )
⇒ AE = `30/2` = 15 cm;
CF = `16/2` = 8 cm
In right ΔOAE,
OA2 = OE2 + AE2
⇒ OE2 = OA2 - AE2 = 172 - 152 = 64
∴ OE = 8 cm
In right ΔOCF,
OC2 = OF2 + CF2
⇒ OF2 = OC2 - CF2 = 172 - 82 = 225
∴ OF = 15 cm
(i) The chord are on the opposite sides of the centre :
∴ EF = EO + OF = 8 + 15 = 23cm
(ii) The chord are on the same side of the centre :
∴ EF = OF - OE = 15 - 8 = 7 cm.
APPEARS IN
संबंधित प्रश्न
A chord of length 6 cm is drawn in a circle of radius 5 cm. Calculate its distance from the centre of the circle.
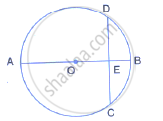
The figure given below, shows a circle with centre O in which diameter AB bisects the chord CD at point E. If CE = ED = 8 cm and EB = 4cm, find the radius of the circle.
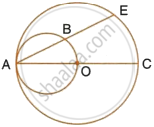
In the given figure, AC is a diameter of a circle, whose centre is O. A circle is described on AO as diameter. AE, a chord of the larger circle, intersects the smaller circle at B. Prove that : AB = BE.
Two chords AB and AC of a circle are equal. Prove that the centre of the circle lies on the bisector of angle BAC.
In the following figure, a circle is inscribed in the quadrilateral ABCD.

If BC = 38 cm, QB = 27 cm, DC = 25 cm and that AD is perpendicular to DC, find the radius of the circle.
The radius of a circle is 13 cm and the length of one of its chords is 24 cm.
Find the distance of the chord from the center.
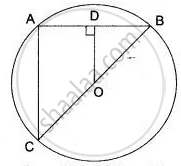
In the given figure, OD is perpendicular to the chord AB of a circle whose center is O. If BC is a diameter, show that CA = 2 OD.
Find the length of a chord which is at a distance of 5 cm from the centre of a circle of radius 13 cm.
AB is a diameter of a circle with centre O and radius OD is perpendicular to AB. If C is any point on arc DB, find ∠ BAD and ∠ ACD.
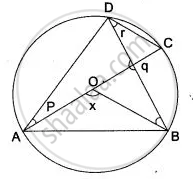
In the figure, AC is the diameter of circle, centre O. Chord BD is perpendicular to AC. Write down the angles p, q, r in term of x.