Advertisements
Advertisements
प्रश्न
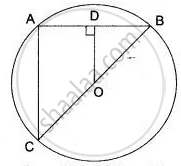
In the given figure, OD is perpendicular to the chord AB of a circle whose center is O. If BC is a diameter, show that CA = 2 OD.
उत्तर
Since OD ⊥ AB and the perpendicular drawn from the centre to a chord bisects the chord.
∴ D is the midpoint of AB.
Also, O being the center is the midpoint of BC.
Thus, in Δ ABC, D and O are the midpoint of AB and BC respectively.
Therefore, OD || AC
and OD = `1/2`CA ....[ ∵ Segment joining the midpoints of two sides of a triangle is half of the third side ]
⇒ CA = 2OD ...Hence proved.
APPEARS IN
संबंधित प्रश्न
A chord of length 8 cm is drawn at a distance of 3 cm from the centre of a circle. Calculate the radius of the circle.
A chord of length 24 cm is at a distance of 5 cm from the centre of the circle. Find the length of the chord of the same circle which is at a distance of 12 cm from the centre.
The figure given below, shows a circle with centre O in which diameter AB bisects the chord CD at point E. If CE = ED = 8 cm and EB = 4cm, find the radius of the circle.
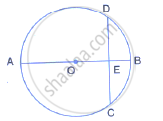
In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other. If ∠MAD = x and ∠BAC = y :
- express ∠AMD in terms of x.
- express ∠ABD in terms of y.
- prove that : x = y.

A chord CD of a circle whose center is O is bisected at P by a diameter AB. Given OA = OB = 15 cm and OP = 9 cm.
Calculate the lengths of: (i) CD ; (ii) AD ; (iii) CB.
A chord of length 6 cm is drawn in a circle of radius 5 cm.
Calculate its distance from the center of the circle.
A chord of length 24 cm is at a distance of 5 cm from the center of the circle. Find the length of the chord of the same circle which is at a distance of 12 cm from the center.
In the given figure, O is the center of the circle with radius 20 cm and OD is perpendicular to AB. If AB = 32 cm,
find the length of CD.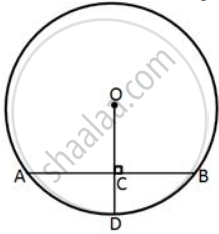
Two chords AB and CD of a circle are parallel and a line L is the perpendicular bisector of AB. Show that L bisects CD.
Find the length of a chord which is at a distance of 5 cm from the centre of a circle of radius 13 cm.
