Advertisements
Advertisements
प्रश्न
In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that:

(ii) angles APB = 90°
उत्तर
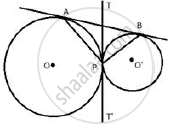
ii) Now in Δ ATP ,
∴ `∠`TAP = `∠`TPA
Similarly in Δ BTP,`∠`TBP = `∠`TPB
Adding,
`∠`TAP +`∠`TBP =`∠`APB
But
∴ TAP + `∠`TBP + `∠`APB =180°
⇒ `∠`APB = `∠`TAP + `∠`TBP =90°
APPEARS IN
संबंधित प्रश्न
Two circles of radii 5 cm and 3 cm are concentric. Calculate the length of a chord of the outer circle which touches the inner.
Radii of two circles are 6.3 cm and 3.6 cm. State the distance between their centres if:
- they touch each other externally,
- they touch each other internally.
In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that :

- tangent at point P bisects AB,
- angles APB = 90°.
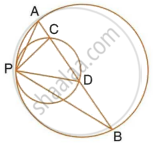
Two circles touch each other internally at a point P. A chord AB of the bigger circle intersects the other circle in C and D. Prove that ∠CPA = ∠DPB.
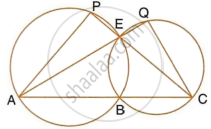
In the given figure; ABC, AEQ and CEP are straight lines. Show that ∠APE and ∠CQE are supplementary.
In the figure, given below, AC is a transverse common tangent to two circles with centres P and Q and of radii 6 cm and 3 cm respectively.
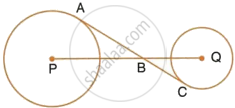
Given that AB = 8 cm, calculate PQ.
Two circles intersect each other at points C and D. Their common tangent AB touches the circles at point A and B. Prove that :
∠ ADB + ∠ ACB = 180°
In which qudrant does point A(-3, 2) lie?
On which axis does point B(12, 0) lie?
Radii of two circles are 6.3 cm and 3.6 cm. State the distance between their centers if -
they touch each other internally.
Two circles with centres O and O' touch each other at point L. Prove that, a tangent through L bisects the common tangent AB of the two circles at point M.

Given: AB is a common tangent of the two circles that touch each other at point L. ML is a tangent through point L.
To prove: M is a mid-point of the tangent AB or MA = MB.
Proof: From the figure,
M is an external point that draws two tangents, MA and ML to the circle with the centre O.
So, `square` = `square` ......(i)
Similarly, M draws two tangents ML and MB to the circle with the centre O'.
So, `square` = `square` ......(ii)
From the equations (i) and (ii),
`square` = `square`
Hence, the tangent at the point L, bisects the common tangent, AB of the two circles at point M.
