Advertisements
Advertisements
प्रश्न
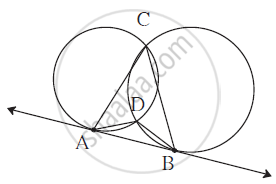
Two circles touch each other internally at a point P. A chord AB of the bigger circle intersects the other circle in C and D. Prove that ∠CPA = ∠DPB.
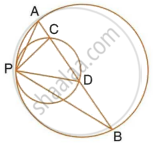
उत्तर
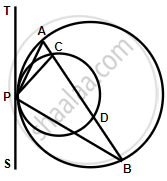
Draw a tangent TS at P to the circles given.
Since TPS is the tangent, PD is the chord.
∴ ∠PAB = ∠BPS ...(i) (Angles in alternate segment)
Similarly,
∠PCD = ∠DPS ...(ii)
Subtracting (i) from (ii)
∠PCD – ∠PAB = ∠DPS – ∠BPS
But in ∠PAC,
Ext. ∠PCD = ∠PAB + ∠CPA
∴ ∠PAB + ∠CPA – ∠PAB = ∠DPS – ∠BPS
`=>` ∠CPA = ∠DPB
APPEARS IN
संबंधित प्रश्न
In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that :

- tangent at point P bisects AB,
- angles APB = 90°.
In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that:

(ii) angles APB = 90°
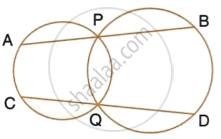
Two circles intersect at P and Q. Through P, a straight line APB is drawn to meet the circles in A and B. Through Q, a straight line is drawn to meet the circles at C and D. Prove that AC is parallel to BD.
Two circles intersect each other at points A and B. Their common tangent touches the circles at points P and Q as shown in the figure. Show that the angles PAQ and PBQ are supplementary.

In the given figure; ABC, AEQ and CEP are straight lines. Show that ∠APE and ∠CQE are supplementary.
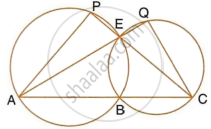
Two circles intersect each other at points C and D. Their common tangent AB touches the circles at point A and B. Prove that :
∠ ADB + ∠ ACB = 180°
In which qudrant does point A(-3, 2) lie?
On which axis does point B(12, 0) lie?
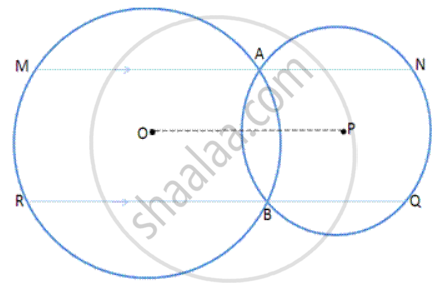
Two circles with centres O and P intersect each other at A and B as shown in following fig. Two straight lines MAN and RBQ are drawn parallel to OP.
Prove that (i) MN = 20 P (ii) MN= RQ.
Radii of two circles are 6.3 cm and 3.6 cm. State the distance between their centers if -
they touch each other internally.
P and Q are the centre of circles of radius 9 cm and 2 cm respectively; PQ = 17 cm. R is the centre of circle of radius x cm, which touches the above circles externally, given that ∠ PRQ = 90°. Write an equation in x and solve it.
