Advertisements
Advertisements
प्रश्न
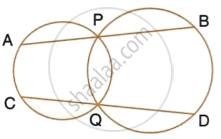
Two circles intersect at P and Q. Through P, a straight line APB is drawn to meet the circles in A and B. Through Q, a straight line is drawn to meet the circles at C and D. Prove that AC is parallel to BD.
उत्तर

Join AC, PQ and BD
ACQP is a cyclic quadrilateral
∴ ∠CAP + ∠PQC = 180° ...(i)
(Pair of opposite in a cyclic quadrilateral are supplementary)
PQDB is a cyclic quadrilateral
∴ ∠PQD + ∠DBP = 180° ...(ii)
(Pair of opposite angles in a cyclic quadrilateral are supplementary)
Again, ∠PQC + ∠PQD = 180° ...(iii)
(CQD is a straight line)
Using (i), (ii) and (iii)
∴ ∠CAP + ∠DBP = 180°
Or ∴ ∠CAB + ∠DBA = 180°
We know, if a transversal intersects two lines such that a pair of interior angles on the same side of the transversal is supplementary, then the two lines are parallel
∴ AC || BD
APPEARS IN
संबंधित प्रश्न
Two circle touch each other internally. Show that the tangents drawn to the two circles from any point on the common tangent are equal in length.
Two circles of radii 5 cm and 3 cm are concentric. Calculate the length of a chord of the outer circle which touches the inner.
Radii of two circles are 6.3 cm and 3.6 cm. State the distance between their centres if:
- they touch each other externally,
- they touch each other internally.
In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that:

(ii) angles APB = 90°
Two parallel tangents of a circle meet a third tangent at points P and Q. Prove that PQ subtends a right angle at the centre.
Two circles touch each other internally at a point P. A chord AB of the bigger circle intersects the other circle in C and D. Prove that ∠CPA = ∠DPB.
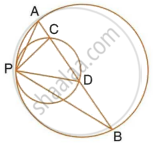
Two circles intersect each other at points A and B. A straight line PAQ cuts the circles at P and Q. If the tangents at P and Q intersect at point T; show that the points P, B, Q and T are concyclic.
Two circles intersect each other at points A and B. Their common tangent touches the circles at points P and Q as shown in the figure. Show that the angles PAQ and PBQ are supplementary.

In which qudrant does point A(-3, 2) lie?
On which axis does point B(12, 0) lie?
Two circles with centres O and O' touch each other at point L. Prove that, a tangent through L bisects the common tangent AB of the two circles at point M.

Given: AB is a common tangent of the two circles that touch each other at point L. ML is a tangent through point L.
To prove: M is a mid-point of the tangent AB or MA = MB.
Proof: From the figure,
M is an external point that draws two tangents, MA and ML to the circle with the centre O.
So, `square` = `square` ......(i)
Similarly, M draws two tangents ML and MB to the circle with the centre O'.
So, `square` = `square` ......(ii)
From the equations (i) and (ii),
`square` = `square`
Hence, the tangent at the point L, bisects the common tangent, AB of the two circles at point M.
