Advertisements
Advertisements
प्रश्न
In a rhombus ABCD, if ∠ACB = 40°, then ∠ADB =
पर्याय
70°
45°
50°
60°
उत्तर
Rhombus ABCD is given as follows:
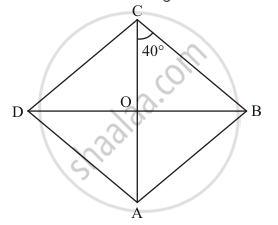
It is given that∠ACB = 40°.
Therefore, ∠OCB = 40° (Because O lies on AC)
We know that the diagonals of a rhombus intersect at right angle.
Therefore, ∠BOC = 90°
By angle sum property of a triangle, we get:
∠CBO + ∠OCB + ∠BOC = 180°
∠CBO + 40° + 90° = 180°
∠CBO+ 130° = 180 °
∠CBO = 50°
Since, O lies on BD
∠CBD = 50°
Also , CB || DA
Therefore,
∠ADB = ∠CBD
∠ADB = 50°
Hence, the correct choice is (c).
APPEARS IN
संबंधित प्रश्न
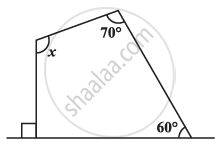
Find the angle measure x in the given Figure
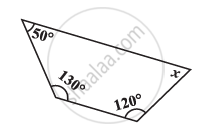
Find the angle measure x in the given Figure
Two opposite angles of a parallelogram are (3x – 2)° and (50 – x)°. Find the measure of each angle of the parallelogram .
Find the measure of all the angles of a parallelogram, if one angle is 24° less than twice the smallest angle
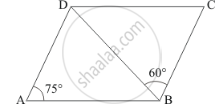
In Fig. below, ABCD is a parallelogram in which ∠DAB = 75° and ∠DBC = 60°. Compute
∠CDB and ∠ADB.
PQRS is a square such that PR and SQ intersect at O. State the measure of ∠POQ.
If PQRS is a square, then write the measure of ∠SRP.
In a quadrilateral ABCD, bisectors of angles A and B intersect at O such that ∠AOB = 75°, then write the value of ∠C + ∠D.
The figure formed by joining the mid-points of the adjacent sides of a square is a
The angle between two altitudes of a parallelogram through the vertex of an obtuse angle of the parallelogram is 60º. Find the angles of the parallelogram.
