Advertisements
Advertisements
प्रश्न
In a survey it was found that 21 people liked product A, 26 liked product B and 29 liked product C. If 14 people liked products A and B, 12 people liked products C and A, 14 people liked products B and C and 8 liked all the three products. Find how many liked product C only.
उत्तर
Let A, B, and C be the set of people who like product A, product B, and product C respectively.
Accordingly, n(A) = 21, n(B) = 26, n(C) = 29, n(A ∩ B) = 14, n(C ∩ A) = 12,
n(B ∩ C) = 14, n(A ∩ B ∩ C) = 8
The Venn diagram for the given problem can be drawn as
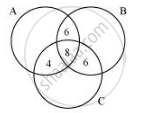
It can be seen that number of people who like product C only is
{29 – (4 + 8 + 6)} = 11
APPEARS IN
संबंधित प्रश्न
Express the truth of each of the following statements by Venn diagram:
(a) Some hardworking students are obedient.
(b) No circles are polygons.
(c) All teachers are scholars and scholars are teachers.
Draw Venn diagram for the truth of the following statements :
Some rectangles are squares.
If A and B are two set such that \[A \subset B\]then find:
\[A \cap B\]
If A = {1, 2, 3, 4, 5}, B = {4, 5, 6, 7, 8}, C = {7, 8, 9, 10, 11} and D = {10, 11, 12, 13, 14}, find:
\[B \cup C\]
If A = {1, 2, 3, 4, 5}, B = {4, 5, 6, 7, 8}, C = {7, 8, 9, 10, 11} and D = {10, 11, 12, 13, 14}, find\[B \cup D\]
If A = {1, 2, 3, 4, 5}, B = {4, 5, 6, 7, 8}, C = {7, 8, 9, 10, 11} and D = {10, 11, 12, 13, 14}, find:
\[B \cup C \cup D\]
If A = {1, 2, 3, 4, 5}, B = {4, 5, 6, 7, 8}, C = {7, 8, 9, 10, 11} and D = {10, 11, 12, 13, 14}, find:
\[\left( A \cup D \right) \cap \left( B \cup C \right)\]
Let \[A = \left\{ x: x \in N \right\}, B = \left\{ x: x - 2n, n \in N \right\}, C = \left\{ x: x = 2n - 1, n \in N \right\}\] and D = {x : x is a prime natural number}. Find: \[A \cap D\]
Let \[A = \left\{ x: x \in N \right\}, B = \left\{ x: x - 2n, n \in N \right\}, C = \left\{ x: x = 2n - 1, n \in N \right\}\] and D = {x : x is a prime natural number}. Find: \[B \cap D\]
Let A = {3, 6, 12, 15, 18, 21}, B = {4, 8, 12, 16, 20}, C = {2, 4, 6, 8, 10, 12, 14, 16} and D = {5, 10, 15, 20}. Find: \[A - D\]
Let A = {3, 6, 12, 15, 18, 21}, B = {4, 8, 12, 16, 20}, C = {2, 4, 6, 8, 10, 12, 14, 16} and D = {5, 10, 15, 20}.
Find: \[B - D\]
Let U = {1, 2, 3, 4, 5, 6, 7, 8, 9}, A = {2, 4, 6, 8} and B = {2, 3, 5, 7}. Verify that \[\left( A \cup B \right)' = A' \cap B'\]
- show the sets U, P and P' by the Venn diagram.
- Verify (P')' = P
Represent the union of two sets by Venn diagram for the following.
P = {a, b, c, e, f} Q = {l, m, n, e, b}
Represent the union of two sets by Venn diagram for the following.
Y = {y | y is an odd number between 90 and 100}
Express the truth of each of the following statements using Venn diagrams:
(a) No circles are polygons
(b) Some quadratic equations have equal roots
Express the truth of each of the following statements using Venn diagram.
(1) All teachers are scholars and scholars are teachers.
(2) If a quadrilateral is a rhombus then it is a parallelogram..
From the given diagram find :
A ∪ B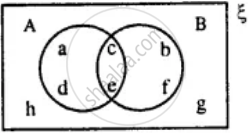
Use the given Venn-diagram to find :
A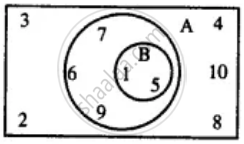
Use the given Venn-diagram to find :
B'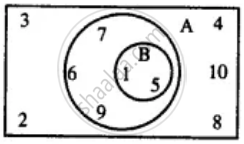
Use the given Venn-diagram to find :
A ∪ B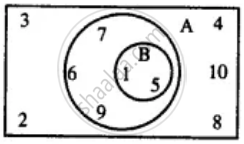
Draw a Venn-diagram to show the relationship between two overlapping sets A and B. Now shade the region representing :
A ∩ B
Draw a Venn-diagram to show the relationship between two overlapping sets A and B. Now shade the region representing :
A ∪ B
Draw a Venn-diagram to show the relationship between two sets A and B; such that A ⊆ B, Now shade the region representing :
B' ∩ A
Two sets A and B are such that A ∩ B = Φ. Draw a venn-diagram to show the relationship between A and B. Shade the region representing :
A ∪ B
Two sets A and B are such that A ∩ B = Φ. Draw a venn-diagram to show the relationship between A and B. Shade the region representing :
B ∩ A'
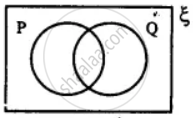
In the given diagram, shade the region which represents the set given underneath the diagrams: (B - A)'

In the given diagram, shade the region which represents the set given underneath the diagrams: (P ∩ Q)'
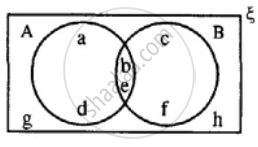
From the given diagram, find :
(i) (A ∪ B) - C
(ii) B - (A ∩ C)
(iii) (B ∩ C) ∪ A
Verify :
A - (B ∩ C) = (A - B) ∪ (A - C)

Using the given diagram, express the following sets in the terms of A and B. {a, d}
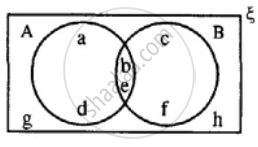
Using the given diagram, express the following sets in the terms of A and B. {a, d, c, f, g, h}
Represent the truth of the following statement by the Venn diagram.
No circles are polygons.
Represent the truth of the following statement by the Venn diagram.
If a quadrilateral is a rhombus, then it is a parallelogram.
Represent the following statement by the Venn diagram.
Some non-resident Indians are not rich.
Represent the following statement by the Venn diagram.
If n is a prime number and n ≠ 2, then it is odd.
Draw the Venn diagrams to illustrate the following relationship among sets E, M and U, where E is the set of students studying English in a school, M is the set of students studying Mathematics in the same school, U is the set of all students in that school.
There is no student who studies both Mathematics and English.
Draw Venn diagram for the following:
Some students are not scholars
