Advertisements
Advertisements
प्रश्न
In the figure, PR || SQ. If PR = 10cm, PT = 5cm, TQ = 6cm and ST = 9cm, calculate RT and SQ.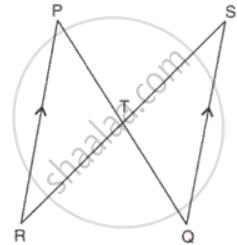
उत्तर
In ΔPRT and ΔSQT
∠PTR = ∠STQ ...(veriically opposite angles)
∠RPT = ∠SQT ...(alternate angles ∵ PR || SQ)
∴ ΔPRT ≅ ΔSQT
⇒ `"RT"/"PT" = "ST"/"TQ"`
⇒ `"RT"/(5) = (9)/(6)`
⇒ RT = `(5 xx 9)/(6)`
⇒ RT = 7.5cm
Also,
`"PT"/"PR" = "TQ"/"SQ"`
⇒ `(5)/(10) = (6)/"SQ"`
⇒ SQ = `(6 xx 10)/(5)`
⇒ SQ = 12cm.
APPEARS IN
संबंधित प्रश्न
The perimeters of two similar triangles ABC and PQR are respectively 36 cm and 24 cm. If PQ = 10 cm, find AB
In figure, if ∠A = ∠C, then prove that ∆AOB ~ ∆COD

The areas of two similar triangles are `81cm^2` and `49cm^2` respectively. If the altitude of the first triangle is 6.3cm, find the corresponding altitude of the other.
In the given figure, A – D – C and B – E – C seg DE || side AB If AD = 5, DC = 3, BC = 6.4 then Find BE.

In the following figure, point D divides AB in the ratio 3 : 5. Find : `(AE)/(AC)`

If ΔABC, D and E are points on AB and AC. Show that DE || BC for each of the following case or not:
AB = 5.6cm, AD = 1.4cm, AC = 7.2cm, and AE = 1.8cm
ΔABC has been reduced by a scale factor 0.6 to ΔA'B'C'/ Calculate: Length of AB, if A'B' = 5.4cm
In ΔABC, AP ⊥ BC and BQ ⊥ AC, B−P−C, A−Q−C, then show that ΔCPA ~ ΔCQB. If AP = 7, BQ = 8, BC = 12, then AC = ?

In ΔCPA and ΔCQB
∠CPA ≅ [∠ ______] ...[each 90°]
∠ACP ≅ [∠ ______] ...[common angle]
ΔCPA ~ ΔCQB ......[______ similarity test]
`"AP"/"BQ" = (["______"])/"BC"` .......[corresponding sides of similar triangles]
`7/8 = (["______"])/12`
AC × [______] = 7 × 12
AC = 10.5
Areas of two similar triangles are equal then prove that triangles are congruent
It is given that ΔABC ~ ΔPQR, with `(BC)/(QR) = 1/3`. Then, `(ar(PRQ))/(ar(BCA))` is equal to ______.
