Advertisements
Advertisements
प्रश्न
In the figure, PR || SQ. If PR = 10cm, PT = 5cm, TQ = 6cm and ST = 9cm, calculate RT and SQ.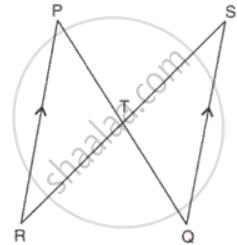
उत्तर
In ΔPRT and ΔSQT
∠PTR = ∠STQ ...(veriically opposite angles)
∠RPT = ∠SQT ...(alternate angles ∵ PR || SQ)
∴ ΔPRT ≅ ΔSQT
⇒ `"RT"/"PT" = "ST"/"TQ"`
⇒ `"RT"/(5) = (9)/(6)`
⇒ RT = `(5 xx 9)/(6)`
⇒ RT = 7.5cm
Also,
`"PT"/"PR" = "TQ"/"SQ"`
⇒ `(5)/(10) = (6)/"SQ"`
⇒ SQ = `(6 xx 10)/(5)`
⇒ SQ = 12cm.
APPEARS IN
संबंधित प्रश्न
In figure, if ∠A = ∠C, then prove that ∆AOB ~ ∆COD

State, true or false:
Two congruent polygons are necessarily similar.
On a map drawn to a scale of 1 : 25000, a triangular plot of a land is marked as ABC with AB= 6cm, BC = 8cm and ∠ ABC = 90° . Calculate the actual length of AB in km and the actual area of the plot in km2 .
A triangle ABC is enlarged, about the point O as centre of enlargement, and the scale factor is 3. Find : BC, if B' C' = 15 cm.
In the following figure, point D divides AB in the ratio 3 : 5. Find : `(AD)/(AB)`

A line segment DE is drawn parallel to base BC of ΔABC which cuts AB at point D and AC at point E. If AB = 5BD and EC = 3.2 cm, find the length of AE.
Through the vertex S of a parallelogram PQRS, a line is drawn to intersect the sides Qp and QR produced at M and N respectively. Prove that `"SP"/"PM" = "MQ"/"QN" = "MR"/"SR"`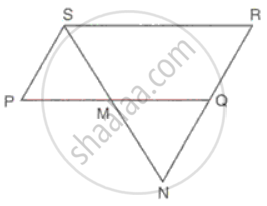
Given is a triangle with sides 3 cm, 5 cm and 6 cm. Find the sides of a triangle which is similar to the given triangle and its shortest side is 4.5 cm.
If BD ⊥ AC and CE ⊥ AB, prove that ∆AEC ~ ∆ADB

D is the mid point of side BC and AE ⊥ BC. If BC = a, AC = b, AB = c, ED = x, AD = p and AE = h, prove that c2 = `"p"^2 - "a"x + "a"^2/4`
