Advertisements
Advertisements
प्रश्न
Through the vertex S of a parallelogram PQRS, a line is drawn to intersect the sides Qp and QR produced at M and N respectively. Prove that `"SP"/"PM" = "MQ"/"QN" = "MR"/"SR"`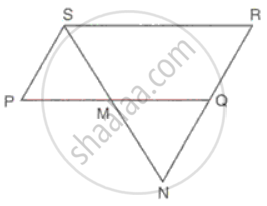
उत्तर
In ΔPMS and ΔMQN
∠PMS = ∠NMQ ...(vertcally oppe angles)
∠SPM = ∠MQN ...(alternate angles, ssince PS || QN)
Therefore, ΔPMS ∼ ΔMQN
∴ `"SP"/"PM" = "MQ"/"QN"` ........(i)
In ΔPMS and ΔMSR
∠PMS = ∠MSR ...(alternate angles, since PM || SR)
SM = SM
Therefore, ΔPMS ∼ ΔMRS
∴ `"SP"/"PM" = "MR"/"SR"` ........(ii)
From (i) and (ii)
∴ `"SP"/"PM" = "MQ"/"QN" = "MR"/"SR"`.
APPEARS IN
संबंधित प्रश्न
In figure, ∠BAC = 90º and segment AD ⊥ BC. Prove that AD2 = BD × DC.
Using Converse of basic proportionality theorem, prove that the line joining the mid-points of any two sides of a triangle is parallel to the third side. (Recall that you have done it in Class IX).
`triangleDEF ~ triangleMNK`. If DE = 5 and MN = 6, then find the value of `(A(triangleDEF))/(A(triangleMNK))`
In ΔPQR, MN is parallel to QR and `(PM)/(MQ) = 2/3`
1) Find `(MN)/(QR)`
2) Prove that ΔOMN and ΔORQ are similar.
3) Find, Area of ΔOMN : Area of ΔORQ

In the given figure, AB || EF || DC; AB = 67.5 cm, DC = 40.5 cm and AE = 52.5 cm.
- Name the three pairs of similar triangles.
- Find the lengths of EC and EF.
In a circle, two chords AB and CD intersect at a point P inside the circle. Prove that
(a) ΔPAC ∼PDB (b) PA. PB= PC.PD

Given that ΔABC ∼ ΔPRQ, name the corresponding angles and the corresponding sides.
Construct a triangle similar to a given triangle PQR with its sides equal to `2/3` of the corresponding sides of the triangle PQR (scale factor `2/3 < 1`)
Two similar triangles will always have ________ angles
In the adjoining diagram the length of PR is ______.

