Advertisements
Advertisements
प्रश्न
Through the vertex S of a parallelogram PQRS, a line is drawn to intersect the sides Qp and QR produced at M and N respectively. Prove that `"SP"/"PM" = "MQ"/"QN" = "MR"/"SR"`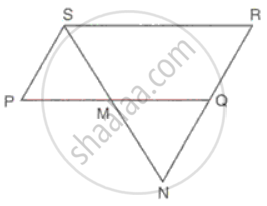
उत्तर
In ΔPMS and ΔMQN
∠PMS = ∠NMQ ...(vertcally oppe angles)
∠SPM = ∠MQN ...(alternate angles, ssince PS || QN)
Therefore, ΔPMS ∼ ΔMQN
∴ `"SP"/"PM" = "MQ"/"QN"` ........(i)
In ΔPMS and ΔMSR
∠PMS = ∠MSR ...(alternate angles, since PM || SR)
SM = SM
Therefore, ΔPMS ∼ ΔMRS
∴ `"SP"/"PM" = "MR"/"SR"` ........(ii)
From (i) and (ii)
∴ `"SP"/"PM" = "MQ"/"QN" = "MR"/"SR"`.
APPEARS IN
संबंधित प्रश्न
In the given figure, two chords AB and CD of a circle intersect each other at the point P (when produced) outside the circle. Prove that
(i) ΔPAC ∼ ΔPDB
(ii) PA.PB = PC.PD

In the given figure, ΔODC~ΔOBA, ∠BOC = 115° and ∠CDO = 700.
Find (i) ∠DCO (ii) ∠DCO (iii) ∠OAB (iv) ∠OBA.

In the given figure, ∠ABC = 90° and BD⊥AC. If AB = 5.7cm, BD = 3.8cm and CD = 5.4cm, find BC.
ΔABC~ΔPQR and ar(ΔABC) = 4, ar(ΔPQR) . If BC = 12cm, find QR.
The areas of two similar triangles are `64cm^2` and `100cm^2` respectively. If a median of the smaller triangle is 5.6cm, find the corresponding median of the other.
As shown in figure, two poles of height 8 m and 4 m are perpendicular to the ground. If the length of shadow of smaller pole due to sunlight is 6 m then how long will be the shadow of the bigger pole at the same time?

A triangle LMN has been reduced by scale factor 0.8 to the triangle L' M' N'. Calculate: the length of M' N', if MN = 8 cm.
A line segment DE is drawn parallel to base BC of ΔABC which cuts AB at point D and AC at point E. If AB = 5BD and EC = 3.2 cm, find the length of AE.
On a map drawn to a scale of 1:25000, a rectangular plot of land has sides 12cm x 16cm. Calculate: The diagonal distance of the plot in km
In the given figure, UB || AT and CU ≡ CB Prove that ΔCUB ~ ΔCAT and hence ΔCAT is isosceles.
