Advertisements
Advertisements
Question
Through the vertex S of a parallelogram PQRS, a line is drawn to intersect the sides Qp and QR produced at M and N respectively. Prove that `"SP"/"PM" = "MQ"/"QN" = "MR"/"SR"`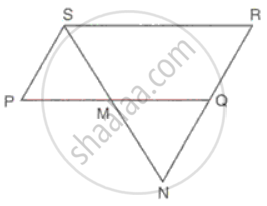
Solution
In ΔPMS and ΔMQN
∠PMS = ∠NMQ ...(vertcally oppe angles)
∠SPM = ∠MQN ...(alternate angles, ssince PS || QN)
Therefore, ΔPMS ∼ ΔMQN
∴ `"SP"/"PM" = "MQ"/"QN"` ........(i)
In ΔPMS and ΔMSR
∠PMS = ∠MSR ...(alternate angles, since PM || SR)
SM = SM
Therefore, ΔPMS ∼ ΔMRS
∴ `"SP"/"PM" = "MR"/"SR"` ........(ii)
From (i) and (ii)
∴ `"SP"/"PM" = "MQ"/"QN" = "MR"/"SR"`.
APPEARS IN
RELATED QUESTIONS
Using Basic proportionality theorem, prove that a line drawn through the mid-points of one side of a triangle parallel to another side bisects the third side. (Recall that you have proved it in Class IX).
In ΔABC and ΔDEF, it is being given that: AB = 5 cm, BC = 4 cm and CA = 4.2 cm; DE=10cm, EF = 8 cm and FD = 8.4 cm. If AL ⊥ BC and DM ⊥ EF, find AL: DM.
ΔABC~ΔDEF and their areas are respectively 64 cm2 and 121cm2. If EF = 15.4cm, find BC.
ΔABC ~ ΔDEF and their areas are respectively `100cm^2` and `49cm2`. If the altitude of ΔABC is 5cm, find the corresponding altitude of ΔDEF.
In the given figure, ABC is a triangle and PQ is a straight line meeting AB in P and AC in Q. If AP = 1cm, PB = 3cm, AQ = 1.5cm, QC = 4.5cm, prove that area of ΔAPQ is 116 of the area of ΔABC.
In a quadrilateral PQRS, the diagonals PR and QS intersect each other at the point T. If PT:TR = QT :TS = 1:2, show that ΔPTQ - DRTS
If figure OPRQ is a square and ∠MLN = 90°. Prove that QR2 = MQ × RN

Areas of two similar triangles are 225 cm2 and 81 cm2. If side of smaller triangle is 12 cm, find corresponding side of major triangle.
There are two poles having heights 8 m and 4 m on plane ground as shown in fig. Because of sunlight shadows of smaller pole is 6m long, then find the length of shadow of longer pole.
An architecture have model of building. Length of building is 1 m then length of model is 0.75 cm. Then find length and height of model building whose actual length is 22.5 m and height is 10 m
