Advertisements
Advertisements
प्रश्न
In the following figure, point D divides AB in the ratio 3 : 5. Find :
- `(AE)/(EC)`
- `(AD)/(AB)`
- `(AE)/(AC)`
Also, if: - DE = 2.4 cm, find the length of BC.
- BC = 4.8 cm, find the length of DE.

उत्तर
i. Given that `(AD)/(DB) = 3/5`
Now, DE is parallel to BC.
Then, by basic proportionality theorem, we have
`(AD)/(DB) = (AE)/(EC)`
`=> (AE)/(EC) = 3/5`
ii. Given that `(AD)/(DB) = 3/5`
So, `(AD)/(AB) = 3/8`
iii. Given that `(AD)/(DB) = 3/5`
So, `(AD)/(AB) = 3/8`
In ΔADE and ΔABC,
∠ADE = ∠ABC ...(Since DE || BC, so the angles are corresponding angles)
∠A = ∠A ...(Common angle)
∴ ΔADE ∼ ΔABC ...(AA criterion for similarity)
`=> (AD)/(AB) = (AE)/(AC)`
`=> (AE)/(AC) = 3/8`
iv. Given that `(AD)/(DB) = 3/5`
So, `(AD)/(AB) = 3/8`
In ΔADE and ΔABC,
∠ADE = ∠ABC ...(Since DE || BC, so the angles are corresponding angles)
∠A = ∠A ...(Common angle)
∴ ΔADE ∼ ΔABC ...(AA criterion for similarity)
`=> (AD)/(AB) = (DE)/(BC)`
`=> 3/8 = (2.4)/(BC)`
`=>` BC = 6.4 cm
v. Given that `(AD)/(DB) = 3/5`
So, `(AD)/(AB) = 3/8`
In ΔADE and ΔABC,
∠ADE = ∠ABC ...(Since DE || BC, so the angles are corresponding angles)
∠A = ∠A ...(Common angle)
∴ ΔADE ∼ ΔABC ...(AA criterion for similarity)
`=> (AD)/(AB) = (DE)/(BC)`
`=> 3/8 = (DE)/(4.8)`
`=>` DE = 1.8 cm
APPEARS IN
संबंधित प्रश्न
The perimeters of two similar triangles ABC and PQR are respectively 36 cm and 24 cm. If PQ = 10 cm, find AB
See the given figure. DE || BC. Find AD.

Given `triangle ABC ~ triangle PQR`, if `(AB)/(PQ) = 1/3`, then find `(ar triangle ABC)/(ar triangle PQR)`
The areas of two similar triangles ABC and PQR are in the ratio 9:16. If BC = 4.5cm, find the length of QR.
In ∆ABC and ∆DEF ∠B = ∠E, ∠F = ∠C and AB = 3DE then which of the statements regarding the two triangles is true ?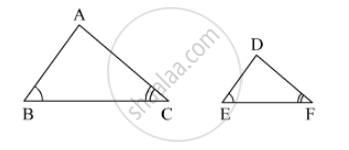
In Δ ABC , MN || BC .

If BC = 14 cm and MN = 6 cm , find `("Ar" triangle "AMN")/("Ar" . ("trapezium MBCN"))`
Construct a triangle similar to a given triangle ABC with its sides equal to `6/5` of the corresponding sides of the triangle ABC (scale factor `6/5 > 1`)
A man whose eye-level is 2 m above the ground wishes to find the height of a tree. He places a mirror horizontally on the ground 20 m from the tree and finds that if he stands at a point C which is 4 m from the mirror B, he can see the reflection of the top of the tree. How height is the tree?
In Quadrilateral ABCD, side AD || BC, diagonal AC and BD intersect in point P, then prove that `"AP"/"PD" = "PC"/"BP"`
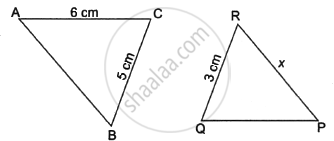
In the given figure, ΔABC ∼ ΔQPR, If AC = 6 cm, BC = 5 cm, QR = 3 cm and PR = x; them the value of x is ______.