Question

O(0, 0), A(6, 0) and B(0, 8) are vertices of a triangle. Find the co-ordinates of the incenter of ∆OAB

Answer 1

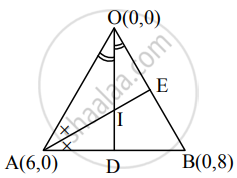
Let bisector of ∠O meet AB at point D and bisector of ∠A meet BO at point E

∴ Point D divides seg AB in the ratio l(OA) : l(OB)

and point E divides seg BO in the ratio l(AB) : l(AO)

Let l be the incentre of ∆OAB.

By distance formula,

l(OA) = `sqrt((0 - 6)^2 + (0 - 0)^2` = 6

l(OB) = `sqrt((0 - 0)^2 + (0 - 8)^2` = 8

∴ Point D divides AB internally in 6 : 8 i.e. 3 : 4

∴ D ≡ `((3(0) + 4(6))/(3 + 4), (3(8) + 4(0))/(3 + 4)) = (24/7, 24/7)`

∴ Equation of OD is `(y - 0)/(24/7 - 0) = (x - 0)/(24/7 - 0)`

∴ y = x    ...(i)

Now, by distance formula,

l(AB) = `sqrt((6 - 0)^2 + (0 - 8)^2`

= `sqrt(36 + 64)`

= 10

l(AO) = `sqrt((6 - 0)^2 + (0 - 0)^2` = 6

∴ Point E divides BO internally in 10 : 6 i.e. 5 : 3

∴ E ≡ `((5(0) + 3(0))/(5 + 3), (5(0) + 3(8))/(5 + 3))` ≡ (0, 3)

∴  Equation of AE is `(y - 0)/(3 - 0) = (x - 6)/(0 - 6)`

∴ `y/3 = (x - 6)/(-6)`

∴ –2y = x – 6

∴ x + 2y = 6    ...(ii)

To find co-ordinates of incentre, we have to solve equations (i) and (ii).

Substituting y = x in (ii), we get

x + 2y = 6

∴ x = 2

Substituting the value of x in (i), we get

y = 2

∴ Co-ordinates of incentre I ≡ (2, 2).

Alternate Method: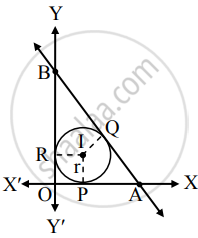

Let I be the incentre.

I lies in the 1^st quadrant

OPIR is a square having side length r.

Since OA = 6, OP = r

PA = 6 – r

Since PA = AQ,

AQ = 6 – r …(i)

Since OB = 8, OR = r,

BR = 8 – r

∴ BR = BQ

∴ BQ = 8 – r …(ii)

AB = BQ + AQ

Also, AB = `sqrt("OA"^2 + "OB"^2)`

= `sqrt(6^2 + 8^2)`

= `sqrt(100)`

= 10

∴ BQ + AQ = 10

∴ (8 – r) + (6 – r) = 10 …[From (i) and (ii)]

∴ 2r = 14 – 10 = 4

∴ r = 2

∴ I = (2, 2)