Advertisements
Advertisements
प्रश्न
Observe all the four triangles FAB, EAB, DAB and CAB as shown in the given figure.

- All triangles have the same base and the same altitude.
- All triangles are congruent.
- All triangles are equal in area.
- All triangles may not have the same perimeter.
उत्तर
1. True.
It is clear from the figure that all triangles have same base AB and all the vertices lie on the same line, so the distance between vertex and base of triangle (i.e. length of altitude) are equal.
2. False
It is clear from the figure that all triangles have only base line is equal and no such other lines are equal to each other.
3. True
Because the triangles on same base and between same parallel lines have equal in area.
4. True
It is clear from the figure that all triangles may not have the same perimeter.
APPEARS IN
संबंधित प्रश्न
ABCD is a rectangle formed by the points A(-1, -1), B(-1, 4), C(5, 4) and D(5, -1). P, Q, R and S are the midpoints of AB, BC, CD and DA respectively. Is the quadrilateral PQRS a square? a rectangle? or a rhombus? Justify your answer.
ΔABC is isosceles with AB = AC = 7.5 cm and BC = 9 cm (see the given figure). The height AD from A to BC, is 6 cm. Find the area of ΔABC. What will be the height from C to AB i.e., CE?

Find the area of the quadrilaterals, the coordinates of whose vertices are
(1, 2), (6, 2), (5, 3) and (3, 4)
Show that the points are the vertices of an isosceles right triangle.

In Figure 1, PS = 3 cm, QS = 4 cm, ∠PRQ = θ, ∠PSQ = 90°, PQ ⊥ RQ and RQ = 9 cm. Evaluate tan θ.
If the co-ordinates of the vertices of an equilateral triangle with sides of length ‘a’ are (x1, y1), (x2, y2), (x3, y3), then `|(x_1, y_1, 1),(x_2, y_2, 1),(x_3, y_3, 1)|^2 = (3"a"^4)/4`
If the points (2, -3), (k, -1), and (0, 4) are collinear, then find the value of 4k.
The area of the triangle whose vertices are A(1, 2), B(-2, 3) and C(-3, -4) is ______.
The area of ∆ABC is 8 cm2 in which AB = AC = 4 cm and ∠A = 90º.
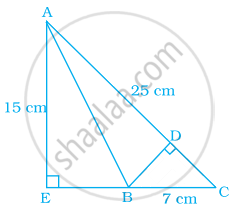
In the given figure, triangle AEC is right-angled at E, B is a point on EC, BD is the altitude of triangle ABC, AC = 25 cm, BC = 7 cm and AE = 15 cm. Find the area of triangle ABC and the length of DB.