Advertisements
Advertisements
प्रश्न
P is a point on the bisector of ∠ABC. If the line through P, parallel to BA meet BC at Q, prove that BPQ is an isosceles triangle.
उत्तर
Given in the question, P is a point on the bisector of ∠ABC. If the line through P, parallel to BA meet BC at Q.
To prove: BPQ is an isosceles triangle.
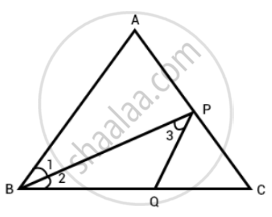
Proof: ∠1 = ∠2 ...(i) [BP is the bisector of ∠ABC]
PQ is parallel to BA and BP cuts them.
So, ∠1 = ∠3 ...[Alternate interior angles as PQ || AB]
∠2 = ∠3 ...[Proved above]
PQ = BQ ...[Sides opposite to equal angle are equal]
Hence, BPQ is an isosceles triangle.
APPEARS IN
संबंधित प्रश्न
If the bisectors of the base angles of a triangle enclose an angle of 135°, prove that the triangle is a right triangle.
In a ΔABC, ∠ABC = ∠ACB and the bisectors of ∠ABC and ∠ACB intersect at O such that ∠BOC = 120°. Show that ∠A = ∠B = ∠C = 60°.
Is the following statement true and false :
All the angles of a triangle can be equal to 60°.
In ΔABC, if bisectors of ∠ABC and ∠ACB intersect at O at angle of 120°, then find the measure of ∠A.
Find the unknown marked angles in the given figure:

Can a triangle together have the following angles?
55°, 55° and 80°
The length of the three segments is given for constructing a triangle. Say whether a triangle with these sides can be drawn. Give the reason for your answer.
17 cm, 7 cm, 8 cm
Can 30°, 60° and 90° be the angles of a triangle?
If an angle of a triangle is equal to the sum of the other two angles, find the type of the triangle
Can we have two acute angles whose sum is a right angle? Why or why not?
