Advertisements
Advertisements
प्रश्न
P is a point on the line joining A(4, 3) and B(–2, 6) such that 5AP = 2BP. Find the co-ordinates of P.
उत्तर
∵ 5AP = 2BP
`\implies (AP)/(BP) = 2/5`
`\implies` AP : PB = 2 : 5
Let the co-ordinates of P be (x, y) which divides the line joining the points A(4, 3) and B(–2, 6) in the ratio of 2 : 5
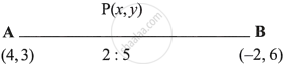
∴ `x = (2 xx (-2) + 5 xx 4)/(2 + 5)`
= `(-4 + 20)/7`
= `16/7`
`y = (2 xx 6 + 5 xx 3)/(2 + 5)`
= `(12 + 15)/7`
= `27/7`
∴ Co-ordinates of P are `(16/7, 27/7)`
APPEARS IN
संबंधित प्रश्न
Prove that the points (–2, –1), (1, 0), (4, 3) and (1, 2) are the vertices of a parallelogram. Is it a rectangle ?
If two vertices of a parallelogram are (3, 2) (-1, 0) and the diagonals cut at (2, -5), find the other vertices of the parallelogram.
Find the distance of the point (1, 2) from the mid-point of the line segment joining the points (6, 8) and (2, 4).
Find the co-ordinates of the points of tri-section of the line joining the points (–3, 0) and (6, 6).
B is a point on the line segment AC. The coordinates of A and B are (2, 5) and (1, 0). If AC= 3 AB, find the coordinates of C.
Show that the line segment joining the points (-3, 10) and (6, -5) is trisected by the coordinates axis.
Find the ratio in which the point P (2, 4) divides the line joining points (-3, 1) and (7, 6).
The perpendicular bisector of the line segment joining the points A(1, 5) and B(4, 6) cuts the y-axis at ______.
If the points A(1, –2), B(2, 3) C(a, 2) and D(– 4, –3) form a parallelogram, find the value of a and height of the parallelogram taking AB as base.
If A and B are (– 2, – 2) and (2, – 4) respectively; then find the co-ordinates of the point P such that `(AB)/(AB) = 3/7`.
