Advertisements
Advertisements
प्रश्न
Points P, Q, and R in that order are dividing line segment joining A (1,6) and B(5, -2) in four equal parts. Find the coordinates of P, Q and R.
उत्तर
The given points are A(1,6) and B(5,-2) .
Then, P (x, y). is a point that devices the line AB in the ratio 1:3
By the section formula:
` x= ((mx_2 +nx_1) /(m+n)) = y = ((my_2+ny_1)/(m+n))`
`⇒ x = (1 xx 5+3xx1)/(1+3) = y = (1 xx(-2) +3xx6)/(1+3)`
` ⇒ x = (5+3)/4 , y = (-2+18)/4`
` ⇒ x = 8/4 , y = 16/4 `
x= 2 and y = 4
Therefore, the coordinates of point P are (2,4)
Let Q be the mid-point of AB
Then, , Q( x y)
` x= (x_1+x_2)/2 , y = (y_1+y_2)/2`
`⇒x = (1+5)/2 , y= (6+(-2))/2`
` ⇒ x =6/2 , y = 4/2 `
⇒ x = 3, y= 2
Therefore, the coordinates of Q are( 3,2)
Let , R( x y) be a point that divides AB in the ratio 3:1
Then, by the section formula:
` x = ((3x_2 +nx_1))/((m+n)) , y = ((my_2 +ny_1))/((m+n))`
` ⇒ x = ((3xx5+1xx1))/(3+1) , y = ((3 xx (-2) +1 xx6))/(3+1)`
`⇒ x = (15+1)/4 , y = (-6+6)/4`
`⇒ x = 16/4 , y= 0/4`
⇒ x = 4 and y = 0
Therefore, the coordinates of R are (4,0) .
Hence, the coordinates of point P, Q and R are (2,4) , (3,2) and (4,0) respectively
APPEARS IN
संबंधित प्रश्न
Two vertices of an isosceles triangle are (2, 0) and (2, 5). Find the third vertex if the length of the equal sides is 3.
A (3, 2) and B (−2, 1) are two vertices of a triangle ABC whose centroid G has the coordinates `(5/3,-1/3)`Find the coordinates of the third vertex C of the triangle.
In what ratio is the line segment joining the points (-2,-3) and (3, 7) divided by the y-axis? Also, find the coordinates of the point of division.
If the point C ( - 2,3) is equidistant form the points A (3, -1) and Bx (x ,8) , find the value of x. Also, find the distance between BC
Show that the points A(3,0), B(4,5), C(-1,4) and D(-2,-1) are the vertices of a rhombus. Find its area.
The base BC of an equilateral triangle ABC lies on y-axis. The coordinates of point C are (0, -3). The origin is the midpoint of the base. Find the coordinates of the points A and B. Also, find the coordinates of another point D such that ABCD is a rhombus.
Points A(-1, y) and B(5,7) lie on the circle with centre O(2, -3y).Find the value of y.
Find the coordinates of the points of trisection of the line segment joining the points (3, –2) and (–3, –4) ?
The points \[A \left( x_1 , y_1 \right) , B\left( x_2 , y_2 \right) , C\left( x_3 , y_3 \right)\] are the vertices of ΔABC .
(i) The median from A meets BC at D . Find the coordinates of the point D.
(ii) Find the coordinates of the point P on AD such that AP : PD = 2 : 1.
(iii) Find the points of coordinates Q and R on medians BE and CF respectively such thatBQ : QE = 2 : 1 and CR : RF = 2 : 1.
(iv) What are the coordinates of the centropid of the triangle ABC ?
If the distance between points (x, 0) and (0, 3) is 5, what are the values of x?
If x is a positive integer such that the distance between points P (x, 2) and Q (3, −6) is 10 units, then x =
If P(2, 4), Q(0, 3), R(3, 6) and S(5, y) are the vertices of a parallelogram PQRS, then the value of y is
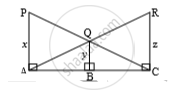
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
Find the point on the y-axis which is equidistant from the points (5, −2) and (−3, 2).
The point R divides the line segment AB, where A(−4, 0) and B(0, 6) such that AR=34AB.">AR = `3/4`AB. Find the coordinates of R.
The line segment joining the points (3, -1) and (-6, 5) is trisected. The coordinates of point of trisection are ______.
Ordinate of all points on the x-axis is ______.
If the perpendicular distance of a point P from the x-axis is 5 units and the foot of the perpendicular lies on the negative direction of x-axis, then the point P has ______.
Which of the points P(0, 3), Q(1, 0), R(0, –1), S(–5, 0), T(1, 2) do not lie on the x-axis?
The distance of the point (–4, 3) from y-axis is ______.
