Advertisements
Advertisements
प्रश्न
PQRS is a parallelogram. T is the mid-point of PQ and ST bisects ∠PSR.
Prove that: QR = QT
उत्तर

∠PST = ∠TSR ............(i)
∠PTS = ∠TSR ............(ii)(alternate angles ∵ SR || PQ)
From (i) and (ii)
∠PST = ∠PTS
Therefore,
PT = PS
But PT = QT ...(T is midpoint of PQ)
And PS = QR ...(PS and QR are opposite and equal sides of a parallelogram)
Hence,
QT = QR.
APPEARS IN
संबंधित प्रश्न
E is the mid-point of side AB and F is the mid-point of side DC of parallelogram ABCD. Prove that AEFD is a parallelogram.
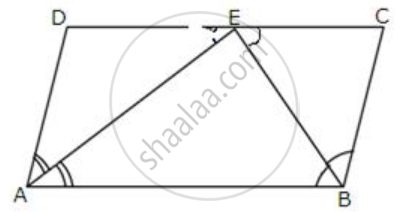
In the given figure, ABCD is a parallelogram.
Prove that: AB = 2 BC.
Points M and N are taken on the diagonal AC of a parallelogram ABCD such that AM = CN. Prove that BMDN is a parallelogram.
PQRS is a parallelogram. T is the mid-point of PQ and ST bisects ∠PSR.
Prove that: ∠RTS = 90°
In a parallelogram ABCD, E is the midpoint of AB and DE bisects angle D. Prove that: BC = BE.
In a parallelogram ABCD, E is the midpoint of AB and DE bisects angle D. Prove that:CE is the bisector of angle C and angle DEC is a right angle
Find the perimeter of the parallelogram PQRS.

In parallelogram ABCD of the accompanying diagram, line DP is drawn bisecting BC at N and meeting AB (extended) at P. From vertex C, line CQ is drawn bisecting side AD at M and meeting AB (extended) at Q. Lines DP and CQ meet at O. Show that the area of triangle QPO is `9/8` of the area of the parallelogram ABCD
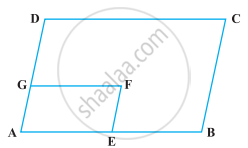
In the following figure, ABCD and AEFG are two parallelograms. If ∠C = 55º, determine ∠F.
Construct a parallelogram POUR in which, PO = 5.5 cm, OU = 7.2 cm and ∠O = 70°.
