Advertisements
Advertisements
प्रश्न
Points M and N are taken on the diagonal AC of a parallelogram ABCD such that AM = CN. Prove that BMDN is a parallelogram.
उत्तर १
Points M are N taken on the diagonal AC of a parallelogram ABCD such that.
Prove that BMDN is a parallelogram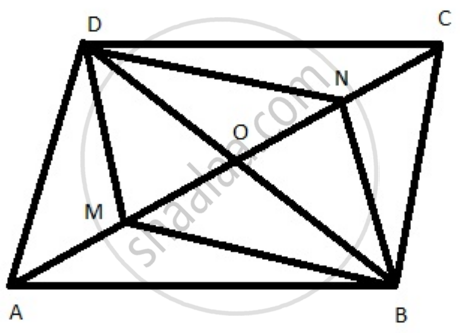
construction: Join B to D to meet AC in O.
Proof: We know that the diagonals of a parallelogram bisect each other.
Now, AC and BD bisect each other at O.
OC = OA
AM = CN
OA - AM = OC - CN
OM = ON
Thus in a quadrilateral BMDN, diagonal BD and MN are such that OM = ON and OD = OB
Therefore the diagonals AC and PQ bisect each other.
Hence BMDN is a parallelogram
उत्तर २
Join BD.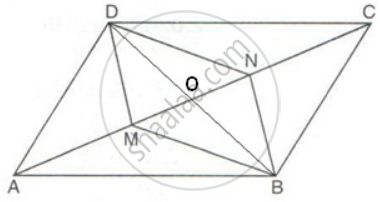
The diagonals of a parallelogram bisect each other.
Therefore, AC and BD bisect each other.
⇒ OA = OC
But AM = CN
Therefore,OA - AM = OC - CN
⇒ OM = ON
Therefore, in quadrilateral BMDN,
OM = ON and OD = OB
⇒ Diagonals MN and BD bisect each other
⇒ BMDN is a parallelogram.
APPEARS IN
संबंधित प्रश्न
In the alongside diagram, ABCD is a parallelogram in which AP bisects angle A and BQ bisects angle B.

Prove that:
- AQ = BP
- PQ = CD
- ABPQ is a parallelogram.
Prove that the bisectors of opposite angles of a parallelogram are parallel.
The following figure shows a trapezium ABCD in which AB is parallel to DC and AD = BC. 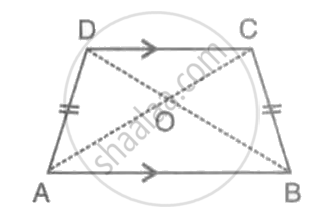
Prove that:
(i) ∠DAB = ∠CBA
(ii) ∠ADC = ∠BCD
(iii) AC = BD
(iv) OA = OB and OC = OD.
In parallelogram ABCD, the bisector of angle A meets DC at P and AB = 2 AD.
Prove that:
(i) BP bisects angle B.
(ii) Angle APB = 90o.
PQRS is a parallelogram. T is the mid-point of PQ and ST bisects ∠PSR.
Prove that: QR = QT
ABCD is a parallelogram. The bisector of ∠BAD meets DC at P, and AD is half of AB.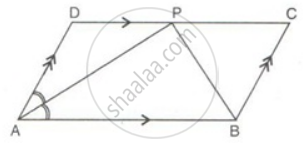
Prove that: BP bisects ∠ABC.
In the given figure, the perimeter of parallelogram PQRS is 42 cm. Find the lengths of PQ and PS.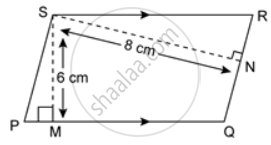
Find the perimeter of the parallelogram PQRS.

In the following figure, it is given that BDEF and FDCE are parallelograms. Can you say that BD = CD? Why or why not?

In the following figure, ABCD and AEFG are two parallelograms. If ∠C = 55º, determine ∠F.