Advertisements
Advertisements
प्रश्न
Prove that the bisectors of opposite angles of a parallelogram are parallel.
उत्तर
Given ABCD is a parallelogram. The bisectors of ∠ADC and ∠BCD meet at E. The bisectors of ∠ABC and ∠BCD meet at F
From the parallelogram ABCD we have
∠ADC + ∠BCD = 180° ...[ sum of adjacent angles of a parallalogram ]
⇒ `("∠ADC")/(2) + ("∠BCD")/(2)` = 90°
⇒ ∠EDC + ∠EDC = 90°
In triangle ECD sum of angles = 180°
⇒ ∠EDC + ∠ECD + ∠CED = 180°
= ∠CED = 90°
Similarly taking triangle BCF it can prove that ∠BFC = 90°
Now since
∠BFC = ∠CED = 90°
Therefore the lines DE and BF are parallel
Hence proved
APPEARS IN
संबंधित प्रश्न
E is the mid-point of side AB and F is the mid-point of side DC of parallelogram ABCD. Prove that AEFD is a parallelogram.
In the alongside diagram, ABCD is a parallelogram in which AP bisects angle A and BQ bisects angle B.

Prove that:
- AQ = BP
- PQ = CD
- ABPQ is a parallelogram.
In the given figure, ABCD is a parallelogram.
Prove that: AB = 2 BC.
In parallelogram ABCD, the bisector of angle A meets DC at P and AB = 2 AD.
Prove that:
(i) BP bisects angle B.
(ii) Angle APB = 90o.
PQRS is a parallelogram. T is the mid-point of PQ and ST bisects ∠PSR.
Prove that: ∠RTS = 90°
In a parallelogram ABCD, E is the midpoint of AB and DE bisects angle D. Prove that: BC = BE.
In the given figure, the perimeter of parallelogram PQRS is 42 cm. Find the lengths of PQ and PS.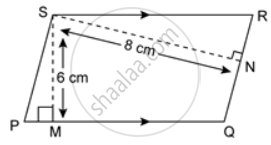
Find the perimeter of the parallelogram PQRS.

In the Figure, ABCD is a rectangle and EFGH is a parallelogram. Using the measurements given in the figure, what is the length d of the segment that is perpendicular to `bar("HE")` and `bar("FG")`?
Which of the following statement is correct?
