Advertisements
Advertisements
प्रश्न
Prove that the common chord of two intersecting circles is bisected at right angles by the line of centres.
उत्तर
Given: Two interesting circles with centres C &D.
AB is their common chord.
To prove: AB bisected by CD at right angles.
Proof : CA = CB ...(radii)
∴ C lies on the right bisector of AB.
Similarly, D lies on the right bisector of AB.
Therefore, CD is the right bisector of AB.
Hence proved.
APPEARS IN
संबंधित प्रश्न
Construct a triangle ABC, in which AB = 4.2 cm, BC = 6.3 cm and AC = 5 cm. Draw perpendicular bisector of BC which meets AC at point D. Prove that D is equidistant from B and C.
In triangle LMN, bisectors of interior angles at L and N intersect each other at point A. Prove that:
- Point A is equidistant from all the three sides of the triangle.
- AM bisects angle LMN.
The given figure shows a triangle ABC in which AD bisects angle BAC. EG is perpendicular bisector of side AB which intersects AD at point F.
Prove that:
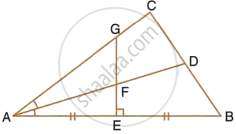
F is equidistant from AB and AC.
Draw an angle ABC = 75°. Draw the locus of all the points equidistant from AB and BC.
Describe the locus of points at distances less than or equal to 2.5 cm from a given point.
Describe the locus of points at distances greater than or equal to 35 mm from a given point.
By actual drawing obtain the points equidistant from lines m and n; and 6 cm from a point P, where P is 2 cm above m, m is parallel to n and m is 6 cm above n.
In Δ ABC, the perpendicular bisector of AB and AC meet at 0. Prove that O is equidistant from the three vertices. Also, prove that if M is the mid-point of BC then OM meets BC at right angles.
In a quadrilateral PQRS, if the bisectors of ∠ SPQ and ∠ PQR meet at O, prove that O is equidistant from PS and QR.
The bisectors of ∠B and ∠C of a quadrilateral ABCD intersect in P. Show that P is equidistant from the opposite sides AB and CD.
