Advertisements
Advertisements
प्रश्न
Prove that the quadrilateral formed by the bisectors of the angles of a parallelogram is a rectangle.
Advertisements
उत्तर
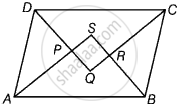
Given: Let ABCD be a parallelogram and AP, BR, CR, be are the bisectors of ∠A, ∠B, ∠C and ∠D, respectively.
To prove: Quadrilateral PQRS is a rectangle.
Proof: Since, ABCD is a parallelogram, then DC || AB and DA is a transversal.
∠A + ∠D = 180° ...[Sum of cointerior angles of a parallelogram is 180°]
⇒ `1/2` ∠A + `1/2` ∠D = 90° ...[Dividing both sides by 2]
∠PAD + ∠PDA = 90°
∠APD = 90° ...[Since, sum of all angles of a triangle is 180°]
∴ ∠SPQ = 90° ...[Vertically opposite angles]
∠PQR = 90°
∠QRS = 90°
And ∠PSR = 90°
Thus, PQRS is a quadrilateral whose each angle is 90°.
Hence, PQRS is a rectangle.
APPEARS IN
संबंधित प्रश्न
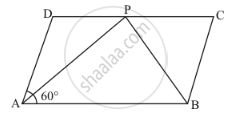
In Fig., below, ABCD is a parallelogram in which ∠A = 60°. If the bisectors of ∠A and ∠B meet at P, prove that AD = DP, PC = BC and DC = 2AD.
In a parallelogram ABCD, if `∠`B = 135°, determine the measures of its other angles .
ABCD is a square. AC and BD intersect at O. State the measure of ∠AOB.
P and Q are the points of trisection of the diagonal BD of a parallelogram AB Prove that CQ is parallel to AP. Prove also that AC bisects PQ.
In a parallelogram ABCD, write the sum of angles A and B.
In a parallelogram ABCD, the bisector of ∠A also bisects BC at X. Find AB : AD.
PQRS is a quadrilateral, PR and QS intersect each other at O. In which of the following case, PQRS is a parallelogram?
∠P = 100°, ∠Q = 80°, ∠R = 95°
We get a rhombus by joining the mid-points of the sides of a
The figure formed by joining the mid-points of the adjacent sides of a rhombus is a
In the given Figure, if AB = 2, BC = 6, AE = 6, BF = 8, CE = 7, and CF = 7, compute the ratio of the area of quadrilateral ABDE to the area of ΔCDF. (Use congruent property of triangles)
