Advertisements
Advertisements
प्रश्न
Show the nature of the following graph for a satellite orbiting the earth.
- KE vs orbital radius R
- PE vs orbital radius R
- TE vs orbital radius R.
उत्तर
Consider the diagram, where a satellite of mass m, moves around the earth in a circular orbit of radius R.

The orbital speed of the satellite orbiting the earth is given by `v_0 = sqrt((GM)/R)` where M and R are the mass and radius of the earth.
a. ∴ KE of a satellite of mass m,
= `1/2 mv_0^2 `
= `1/2m xx (GM)/R`
∴ `E_k ∝ 1/R`
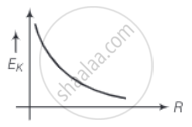
It means the KE decrease exponentially with radius. The graph for KE versus orbital radius R is shown in figure.
b. Potential energy of a satellite `E-p = - (GMn)/R`
`E_p ∝ 1/R`

The graph for PE versus orbital radius R is shown in figure.
c. Total energy of the satellite `E = E_k + E_p`
= `(Gmm)/(2R) - (GMm)/R`
= `- (GMm)/(2R)`
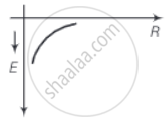
The graph for total energy versus orbital radius R is shown in the figure.
APPEARS IN
संबंधित प्रश्न
A satellite of mass 1000 kg is supposed to orbit the earth at a height of 2000 km above the earth's surface. Find (a) its speed in the orbit, (b) is kinetic energy, (c) the potential energy of the earth-satellite system and (d) its time period. Mass of the earth = 6 × 1024kg.
Answer the following question.
What is periodic time of a geostationary satellite?
Answer the following question.
Why is a minimum two-stage rocket necessary for launching of a satellite?
Solve the following problem.
Calculate the speed of a satellite in an orbit at a height of 1000 km from the Earth’s surface.
(ME = 5.98 × 1024 kg, R = 6.4 × 106 m)
Solve the following problem.
Calculate the value of the universal gravitational constant from the given data. Mass of the Earth = 6 × 1024 kg, Radius of the Earth = 6400 km, and the acceleration due to gravity on the surface = 9.8 m/s2.
A body weighs 5.6 kg wt on the surface of the Earth. How much will be its weight on a planet whose mass is 7 times the mass of the Earth and radius twice that of the Earth’s radius?
Solve the following problem.
What is the gravitational potential due to the Earth at a point which is at a height of 2RE above the surface of the Earth?
(Mass of the Earth is 6 × 1024 kg, radius of the Earth = 6400 km and G = 6.67 × 10–11 N m2 kg–2)
A geostationary satellite is orbiting the earth at the height of 6R above the surface of earth. R being radius of earth. The time period of another satellite at a height of 2.5 R from the surface of earth is ____________.
A satellite is to revolve round the earth in a circle of radius 9600 km. The speed with which this satellite be projected into an orbit, will be ______.
Two satellites are orbiting around the earth in circular orbits of same radius. One of them is 10 times greater in mass than the other. Their period of revolutions are in the ratio ______.
