Advertisements
Advertisements
प्रश्न
State Kepler's law of orbit and law of equal areas.
उत्तर १
Ist law ( Law of orbit ) : The orbital path in the solar system is an ellipse with sun as one focus.
2nd law ( Law of equal area ) : The radius vector joining the centre of the planet to the centre of sun traces out equal area in equal intervals of time.
i.e.The area velocity of the planet is constant
उत्तर २
All planet revolves around the sun in the elliptical orbit, the sun as one of its focus.
The line joining sun and planet sweeps the equal area in equal time interval i.e. Areal velocity is constant.
APPEARS IN
संबंधित प्रश्न
State Kepler's laws of planetary motion.
Let the period of revolution of a planet at a distance R from a star be T. Prove that if it was at a distance of 2R from the star, its period of revolution will be \[\sqrt{8}\] T.
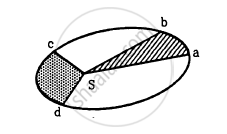
In the Following figure shows the elliptical path of a planet about the sun. The two shaded parts have equal area. If t1 and t2 be the time taken by the planet to go from a to b and from c to d respectively,
Answer the following question.
State Kepler’s law of equal areas.
Answer the following question.
State Kepler’s law of the period.
Answer the following question in detail.
State Kepler’s three laws of planetary motion.
Observe the given figure showing the orbit of a planet moving around the Sun and write the three laws related to it:

The orbit of a planet moving around the Sun
Observe the given figure and answer these following questions.

The orbit of a planet moving around the Sun
- What is the conclusion about the orbit of a planet?
- What is the relation between velocity of planet and distance from sun?
- Explain the relation between areas ASB, CSD and ESF.
Write the Kepler's laws.
The third law of Kepler is also known as the Law of ______.
State Kepler’s laws.
The earth moves around the sun in an elliptical orbit as shown in the figure. The ratio, `"OA"/"OB"` = x. The ratio of the speed of the earth at Band at A is ______.

To verify Kepler's third law graphically four students plotted graphs. Student A plotted a graph of T (period of revolution of planets) versus r (average distance of planets from the sun) and found the plot is straight line with slope 1.85. Student B plotted a graph of T2 v/s r3 and found the plot is straight line with slope 1.39 and negative Y-intercept. Student C plotted graph of log T v/s log r and found the plot is straight line with slope 1.5. Student D plotted graph of log T v/s log r and found the plot is straight line with slope 0.67 and with negative X-intercept. The correct graph is of student
A planet revolves in an elliptical orbit around the sun. The semi-major and minor axes are a and b, then the time period is given by:
If the sun and the planets carried huge amounts of opposite charges ______.
- all three of Kepler’s laws would still be valid.
- only the third law will be valid.
- the second law will not change.
- the first law will still be valid.
Draw areal velocity versus time graph for mars.
What is the direction of areal velocity of the earth around the sun?
A star like the sun has several bodies moving around it at different distances. Consider that all of them are moving in circular orbits. Let r be the distance of the body from the centre of the star and let its linear velocity be v, angular velocity ω, kinetic energy K, gravitational potential energy U, total energy E and angular momentum l. As the radius r of the orbit increases, determine which of the above quantities increase and which ones decrease.
A satellite is in an elliptic orbit around the earth with aphelion of 6R and perihelion of 2 R where R= 6400 km is the radius of the earth. Find eccentricity of the orbit. Find the velocity of the satellite at apogee and perigee. What should be done if this satellite has to be transferred to a circular orbit of radius 6R ?
[G = 6.67 × 10–11 SI units and M = 6 × 1024 kg]
The maximum and minimum distances of a comet from the Sun are 1.6 × 1012 m and 8.0 × 1010 m respectively. If the speed of the comet at the nearest point is 6 × 104 ms-1, the speed at the farthest point is ______.
lf the angular momentum of a planet of mass m, moving around the Sun in a circular orbit is L, about the center of the Sun, and its areal velocity is ______.
Two planets A and B of equal mass are having their period of revolutions TA and TB such that TA = 2TB. These planets are revolving in the circular orbits of radii rA and rB respectively. Which out of the following would be the correct relationship of their orbits?
