Advertisements
Advertisements
प्रश्न
Suppose that the radius of cross-section of the wire used in the previous problem is r. Find the increase in the radius of the loop if the magnetic field is switched off. Young's modulus of the material of the wire is Y.
उत्तर
Given:
Radius of cross-section of the wire = r
Young's modulus of the material of the wire is Y.
As per the question, when the applied magnetic field is switched off, the tension in the wire increased and so did its length.
Young's modulus,
`Y =(stress)/"strain"`
`Y=(T//A)/(Deltal//l)`
Here,
T is the tension
A is the area of cross-section
Δl is the increse in length of the wire
`Deltal = (Tl)/(YA) = (iaBxx1)/(Yxxpir^2)`
`2pixx Deltar = (iaBxx2pia)/(Yxxpir^2)`
`Delta r =( ia^2B)/(pir^2Y)`
APPEARS IN
संबंधित प्रश्न
Draw a neat and labelled diagram of suspended coil type moving coil galvanometer.
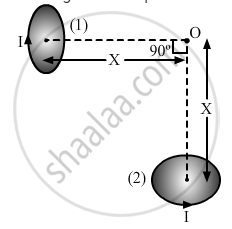
Two very small identical circular loops, (1) and (2), carrying equal currents I are placed vertically (with respect to the plane of the paper) with their geometrical axes perpendicular to each other as shown in the figure. Find the magnitude and direction of the net magnetic field produced at the point O.
Will a current loop placed in a magnetic field always experience a zero force?
A rectangular wire-loop of width a is suspended from the insulated pan of a spring balance, as shown in the figure. A current i exists in the anti-clockwise direction in the loop. A magnetic field B exists in the lower region. Find the change in the tension of the spring if the current in the loop is reversed.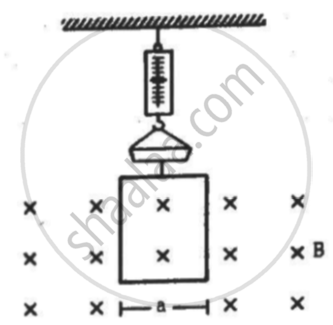
A rectangular loop of sides 20 cm and 10 cm carries a current of 5.0 A. A uniform magnetic field of magnitude 0.20 T exists parallel to the longer side of the loop. (a) What is the force acting on the loop? (b) What is the torque acting on the loop?
A circular loop carrying a current i is made of a wire of length L. A uniform magnetic field B exists parallel to the plane of the loop. (a) Find the torque on the loop. (b) If the same length of the wire is used to form a square loop, what would be the torque? Which is larger?
Derive the expression for the torque acting on a current-carrying loop placed in a magnetic field.
Derive the expression for the torque on a current-carrying coil in a magnetic field.
A small cylindrical soft iron piece is kept in a galvanometer so that
If number of turns in moving coil galvanometer becomes half, then the deflection for the same current will become ____________.
The sensitivity of moving coil galvanometer is inversely proportional to ____________.
Which one of the following statements is 'NOT' TRUE? Sensitivity of a moving coil galvanometer can be increased by ____________.
The magnetic field developed due to current carrying coil at its centre is 'B'. If the new coil of two turns is prepared from the above coil and same current is passed, then the magnetic field at the centre of the new coil will be ____________.
A circular coil having N turns and radius r carries a current I. It is held in the XZ plane in a magnetic field `Bhati`. The torque on the coil due to the magnetic field is ______.
A current of 10 A is flowing in a wire of length 1.5 m. A force of 15 N acts on it when it is placed in a uniform magnetic field of 2 T. The angle between the magnetic field and the direction of the current is ______.
Calculate the orbital magnetic dipole moment of the electron in the second Bohr orbit of the hydrogen atom, given the radius of the orbit is 2.1 Å and the orbital speed is 1.1 × 106 m/s.
Write the formula for torque acting on rotating current carrying coil in terms of magnetic dipole moment, in vector form.
An electron moving along positive X axis with a velocity of 8 ×107ms-1 enters a region having uniform magnetic field B = 1.3 × 10-3 T along positive Y axis.
- Explain why the electron describes a circular path.
- Calculate the radius of the circular path described by the electron.
