Advertisements
Advertisements
प्रश्न
The area of circle is equal to the sum of the areas of two circles of radii 24 cm and 7 cm. The diameter of the new circle is
पर्याय
25 cm
31 cm
50 cm
62 cm
उत्तर
50 cm
Let r cm be the radius of the new circle.
Now,
Area of the new circle = Area of the circle with radius 24 cm + Area of the circle with radius 7 cm
Thus, we have:
`πr^2 = pi"r"_1^2 + pi"r"_2^2`
`=> pi"r"^2=[pixx(24)^2+pixx(7)^2]"cm"^2`
`=>pi"r"^2 = [pixx576+pixx49]"cm"^2`
`=>pi"r"^2 = pixx(576+49) "cm"^2`
`=> "r"^2 = 625 pi "cm"^2`
`=>"r"^2 = 625`
`=> "r" = 25`
∴ Diameter of the new circle = (25 × 2) cm
= 50 cm
APPEARS IN
संबंधित प्रश्न
Find the area of the quadrilateral ABCD in which AD = 24 cm, ∠BAD 90° and ∠BCD is an equilateral triangle having each side equal to 26 cm. Also, find the perimeter of the quadrilateral.

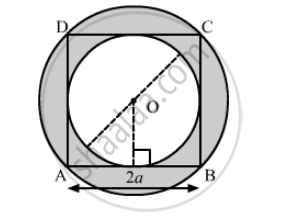
In the following figure, ABCD is a square of side 2a, Find the ratio between
(i) the circumferences
(ii) the areas of the in circle and the circum-circle of the square.
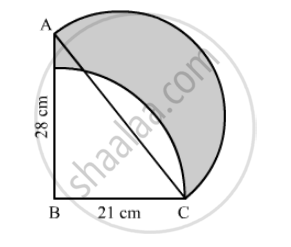
In the following figure, ABC is a right-angled triangle, ∠B = 90°, AB = 28 cm and BC = 21 cm. With AC as diameter a semicircle is drawn and with BC as radius a quarter circle is drawn. Find the area of the shaded region correct to two decimal places.
In the following figure, ABCD is a trapezium of area 24.5 cm2 , If AD || BC, ∠DAB = 90°, AD = 10 cm, BC = 4 cm and ABE is quadrant of a circle, then find the area of the shaded region. [CBSE 2014]

The radius of a circle is 20 cm. It is divided into four parts of equal area by drawing three concentric circles inside it. Then, the radius of the largest of three concentric circles drawn is
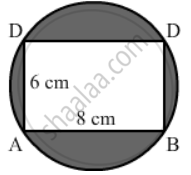
In the given figure, ABCD is a rectangle inscribed in a circle having length 8 cm and breadth 6 cm. If π = 3.14, then the area of the shaded region is:
Find the radius and area of a circle, whose circumference is :
(i) 132 cm
(ii) 22 m
There is a path of uniform width 7 m round and outside a circular garden of diameter 210 m. Find the area of the path.
A 7m wide road surrounds a circular garden whose area is 5544m2. Find the area of the road and the cost of tarring it at the rate of Rs.150 per m2.
Area of a rectangle and the area of a circle are equal. If the dimensions of the rectangle are 14 cm × 11 cm, then radius of the circle is ______.
