Advertisements
Advertisements
प्रश्न
The areas of two similar triangles are 81 cm2 and 49 cm2 respectively. Find the ratio of their corresponding heights. What is the ratio of their corresponding medians?
उत्तर
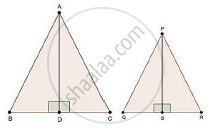
We have,
ΔABC ~ ΔPQR
Area (ΔABC) = 81 cm2,
Area (ΔPQR) = 49 cm2
And AD and PS are the altitudes
By area of similar triangle theorem
`("Area"(triangleABC))/("Area"(trianglePQR))="AB"^2/"PQ"^2`
`rArr81/49="AB"^2/"PQ"^2`
`rArr9/7="AB"/"PQ"` ….(i) [Taking square root]
𝐼𝑛 ΔABD and ΔPQS
∠B = ∠Q [ΔABC ~ ΔPQR]
∠ ADB = ∠PSQ [Each 90°]
Then, ΔABD ~ ΔPQS [By AA similarity]
`therefore"AB"/"PQ"="AD"/"PS"` …(ii) [Corresponding parts of similar Δ are proportional]
Compare (1) and (2)
`"AD"/"PS"=9/7`
∴ Ratio of altitudes = 9/7
Since, the ratio of the area of two similar triangles is equal to the ratio of the squares of the squares of their corresponding altitudes and is also equal to the squares of their corresponding medians.
Hence, ratio of altitudes = Ratio of medians = 9 : 7
APPEARS IN
संबंधित प्रश्न
Diagonals of a trapezium ABCD with AB || DC intersect each other at the point O. If AB = 2CD, find the ratio of the areas of triangles AOB and COD.
D, E and F are respectively the mid-points of sides AB, BC and CA of ΔABC. Find the ratio of the area of ΔDEF and ΔABC.
Triangles ABC and DEF are similar If AC = 19cm and DF = 8 cm, find the ratio of the area of two triangles.
Triangles ABC and DEF are similar If area (ΔABC) = 36 cm2, area (ΔDEF) = 64 cm2 and DE = 6.2 cm, find AB.
Triangles ABC and DEF are similar If AB = 1.2 cm and DE = 1.4 cm, find the ratio of the areas of ΔABC and ΔDEF.
In ΔABC, PQ is a line segment intersecting AB at P and AC at Q such that seg PQ || seg BC. If PQ divides ΔABC into two equal parts having equal areas, find `"BP"/"AB"`.
If ΔABC is similar to ΔDEF such that 2 AB = DE and BC = 8 cm then EF is equal to ______.
In ΔLMN, ∠L = 50° and ∠N = 60°, If ΔLMN ~ ΔPQR, then find ∠Q.
In the given figure, D is the mid-point of BC, then the value of `(coty^circ)/(cotx^circ)` is ______.
If ΔABC ∼ ΔPQR and `(A(ΔABC))/(A(ΔPQR)) = 16/25`, then find AB : PQ.
