Advertisements
Advertisements
प्रश्न
The bisectors of base angles of a triangle cannot enclose a right angle in any case.
उत्तर
In a ABC
Sum of all angles of triangles is `180^@`
i.e, `∠ A+∠ B+∠C =180^@` divide both sides by `2`
⇒ `1/2∠A+1/2∠B+1/2∠C=180^@`
⇒`1/2∠A+∠OBC+∠OBC=90^@` [∵ OB,OC insects ∠B and ∠C]
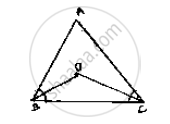
⇒`∠OBC+∠OCB=90^@-1/2A`
Now in `Δ OCB=180^@`
`∴ ∠ BOC+∠OBC+∠OCB=180^@`
⇒`∠ BOC+90^@-1/2∠A=180^@`
⇒ `∠ BOC=90^@-1/2 ∠A`
Hence, bisectors of a base angle cannot enclose right angle.
APPEARS IN
संबंधित प्रश्न
Define a triangle.
Mark the correct alternative in each of the following:
If all the three angles of a triangle are equal, then each one of them is equal to
An exterior angle of a triangle is equal to 100° and two interior opposite angles are equal. Each of these angles is equal to
Side BC of a triangle ABC has been produced to a point D such that ∠ACD = 120°. If ∠B = \[\frac{1}{2}\]∠A is equal to
Calculate the unknown marked angles of the following figure :
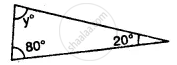
In the following, find the marked unknown angle:

Can a triangle together have the following angles?
33°, 74° and 73°
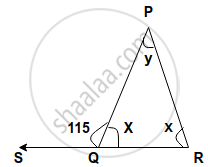
Find, giving a reason, the unknown marked angles, in a triangle drawn below:
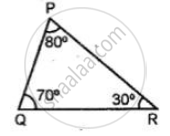
Classify the following triangle according to angle:
The number of angles in figure is ______.
