Advertisements
Advertisements
प्रश्न
The cost of fencing a square lawn at ₹ 14 per meter is ₹ 28000. Find the cost of mowing the lawn at ₹ 54 100 per `m^2`
उत्तर
Cost of fencing the lawn Rs` 28000`
Let lbe the length of each side of the lawn. Then, the perimeter is 4. l We know
`"Cost"= "Rate"xx "Perimeter"`
⇒`28000=14 xx41`
⇒` 28000=56l`
Or,` l=28000/56=500m`
Area of the square lawn=`500xx500=250000 m^2`
Cost of moving `100 m^2` of the lawn= `Rs 54`
Cost of moving `1m^2` of the lawn=Rs `54/100`
∴ Cost of moving `250000 m^2` of the lawn =` (250000xx54)/100`= `Rs 135000`
APPEARS IN
संबंधित प्रश्न
The adjacent sides of a parallelogram are 32 cm and 24 cm. If the distance between the longer sides is 17.4 cm, find the distance between the shorter sides.
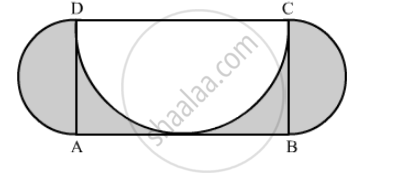
In the following figure, ABCD is a rectangle with AB = 14 cm and BC = 7 cm. Taking DC, BC and AD as diameters, three semi-circles are drawn as shown in the figure. Find the area of the shaded region.
What is the angle subtended at the centre of a circle of radius 6 cm by an arc of length 3 π cm?
If the area of a square is same as the area of a circle, then the ratio of their perimeters, in terms of π, is
If the area of the circle is numerically equal to twice its circumference then what is the diameter of the circle?
ABCD is a field in the shape of a trapezium, AD || BC, ∠ABC = 90° and ∠ADC = 60°. Four sectors are formed with centres A, B, C and D, as shown in the figure. The radius of each sector is 14 m. Find the following:
- total area of the four sectors,
- area of the remaining portion, given that AD = 55 m, BC = 45 m and AB = 30 m.

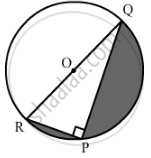
In the given figure, PQ = 24, PR = 7 cm and O is the centre of the circle. Find the area of the shaded region.
The diameter of a wheel is 40 cm. How many revolutions will it make in covering 176 m?
A circular field of radius 105 m has a circular path of uniform width of 5 m along and inside its boundary. Find the area of the path.
The area of the circle whose diameter is 21 cm is ____________.
