Advertisements
Advertisements
Question
The cost of fencing a square lawn at ₹ 14 per meter is ₹ 28000. Find the cost of mowing the lawn at ₹ 54 100 per `m^2`
Solution
Cost of fencing the lawn Rs` 28000`
Let lbe the length of each side of the lawn. Then, the perimeter is 4. l We know
`"Cost"= "Rate"xx "Perimeter"`
⇒`28000=14 xx41`
⇒` 28000=56l`
Or,` l=28000/56=500m`
Area of the square lawn=`500xx500=250000 m^2`
Cost of moving `100 m^2` of the lawn= `Rs 54`
Cost of moving `1m^2` of the lawn=Rs `54/100`
∴ Cost of moving `250000 m^2` of the lawn =` (250000xx54)/100`= `Rs 135000`
APPEARS IN
RELATED QUESTIONS
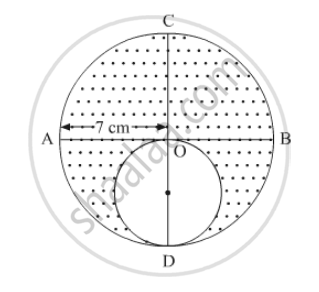
In the following figure, AB and CD are two diameters of a circle perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region.
In the following figure, ABCD is a trapezium of area 24.5 cm2 , If AD || BC, ∠DAB = 90°, AD = 10 cm, BC = 4 cm and ABE is quadrant of a circle, then find the area of the shaded region. [CBSE 2014]

In a circle of radius 10 cm, an arc subtends an angle of 108° at the centre. what is the area of the sector in terms of π?
Write the formula for the area of a sector of angle \[\theta\] (in degrees) of a circle of radius r.
If the radius of a circle is diminished by 10%, then its area is diminished by
ABCD is a rectangle whose three vertices are B (4,0), C (4,3) and D (0, 3). The length of one of its diagonals is
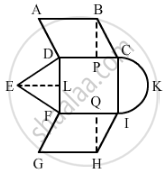
A child draws the figure of an aeroplane as shown. Here, the wings ABCD and FGHI are parallelograms, the tail DEF is an isosceles triangle, the cockpit CKI is a semicircle and CDFI is a square. In the given figure, BP ⊥ CD, HQ ⊥ FI and EL ⊥ DF. If CD = 8 cm, BP = HQ = 4 cm and DE = EF = 5 cm, find the area of the whole figure.
The area of the sector of angle θ° of a circle with radius R is
A chord of a circle of radius 14 cm a makes a right angle at the centre. Find the area of the sector.
The area of a circle is 1386 sq.cm; find its circumference.
