Advertisements
Advertisements
प्रश्न
The cross section of a piece of metal 2 m in length
is shown. Calculate the volume of the piece of metal.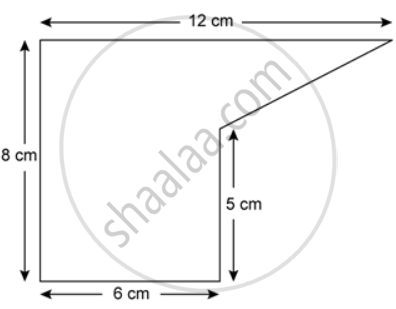
उत्तर

Length (height) of the metal
= 2m
= 200cm
Volume of the metal
= Area of cross-section x height
= 57 x 200
= 11400cm3
∴ Volume of the metal is 11400cm3.
APPEARS IN
संबंधित प्रश्न
The following figure shows a closed victory-stand whose dimensions are given in cm.
Find the volume and the surface area of the victory stand.
A rectangular cardboard sheet has length 32 cm and breadth 26 cm. Squares each of side 3 cm, are cut from the corners of the sheet and the sides are folded to make a rectangular container. Find the capacity of the container formed.
A rectangular water-tank measuring 80 cm x 60 cm is filled form a pipe of cross-sectional area 1.5 cm2, the water emerging at 3.2 m/s. How long does it take to fill the tank?
The cross-section of a piece of metal 4 m in length is shown below. Calculate :
(i) The area of the cross-section;
(ii) The volume of the piece of metal in cubic centimeters.
If 1 cubic centimeter of the metal weighs 6.6 g, calculate the weight of the piece of metal to the nearest kg.
A school auditorium is 40 m long, 30 m broad and 12 m high. If each student requires 1.2 m2 of the floor area; find the maximum number of students that can be accommodated in this auditorium. Also, find the volume of air available in the auditorium, for each student.
Find the length of a solid cylinder of diameter 4 cm when recast into a hollow cylinder of outer diameter 10 cm, thickness 0.25 cm and length 21 cm? Give your answer correct to two decimal places.
The cross section of a piece of metal 2 m in length is shown. Calculate the area of cross section.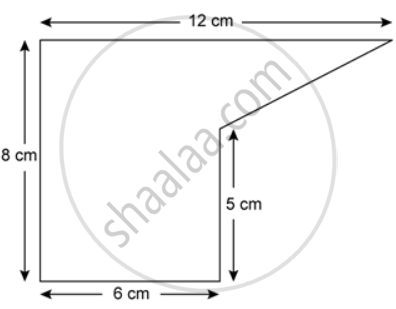
A swimming pool is 50 m long and 15 m wide. Its shallow and deep ends are 1.5 m and 4.5 m respectively. If the bottom of the pool slopes uniformly, find the amount of water in kilolitres required to fill the pool (1 m3 = 1000 liters).
The cross section of a tunnel perpendicular to its length is a trapezium ABCD as shown in the figure. AM = BN; AB = 4.4 m, CD = 3 m The height of a tunnel is 2.4 m. The tunnel is 5.4 m long. Calculate the cost of painting the internal surface of the tunnel (excluding the floor) at the rate of Rs. 5 per m2.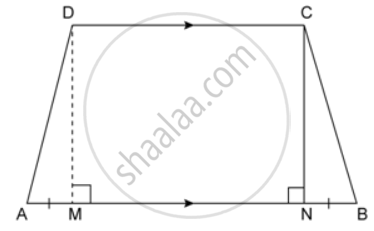
The cross section of a canal is a trapezium with the base length of 3 m and the top length of 5 m. It is 2 m deep and 400 m long. Calculate the volume of water it holds.
