Question

The following figure shows a small spherical ball of mass m rolling down the loop track. The ball is released on the linear portion at a vertical height H from the lowest point. The circular part shown has a radius R.
(a) Find the kinetic energy of the ball when it is at a point A where the radius makes an angle θ with the horizontal.
(b) Find the radial and the tangential accelerations of the centre when the ball is at A.
(c) Find the normal force and the frictional force acting on the if ball if H = 60 cm, R = 10 cm, θ = 0 and m = 70 g.

Answer 1

(a) Let the velocity and angular velocity of the ball at point A be v and ω, respectively.

Total kinetic energy at point A \[= \frac{1}{2}m v^2  + \frac{1}{2}I \omega^2\]

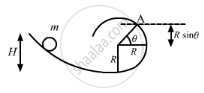
Total potential energy at point A \[= mg\left( R + R\sin\theta \right)\]

On applying the law of conservation of energy, we have

Total energy at initial point = Total energy at A

Therefore, we get

\[mgH = \frac{1}{2}m v^2  + \frac{1}{2}I \omega^2  + mgR\left( 1 + \sin\theta \right)\]

\[ \Rightarrow mgH - mgR\left( 1 + \sin\theta \right) = \frac{1}{2}m \nu^2  + \frac{1}{2}I \omega^2 \]

\[ \Rightarrow \frac{1}{2}m v^2  + \frac{1}{2}I \omega^2  = mg\left( H - R - R\sin\theta \right)........(1)\]

\[\text{Total }K . E .  \text{ at } A = mg\left( H - R - R\sin\theta \right)\]

(b) Let us now find the acceleration components.

Putting \[I = \frac{2}{5}m R^2 \text{ and } \omega = \frac{v}{R}\] in equation (1), we get

\[\frac{7}{10}m v^2  = mg\left( H - R - R\sin\theta \right)\]

\[ \Rightarrow  v^2  = \frac{10}{7}g\left( H - R - R\sin\theta \right).........(2)\]

Radial acceleration,

\[a_r  = \frac{v^2}{R} = \frac{10}{7}\frac{g\left( H - R - R\sin\theta \right)}{R}\]

For tangential acceleration,

Differentiating equation (2) w.r.t. `'t'`,

\[2v\frac{dv}{dt} =  - \left( \frac{10}{7} \right)gR\cos\theta\frac{d\theta}{dt}\]

\[ \Rightarrow \omega R\frac{dv}{dt} =  - \left( \frac{5}{7} \right)  gR\cos\theta\frac{d\theta}{dt}\]

\[ \Rightarrow \frac{dv}{dt} =  - \left( \frac{5}{7} \right)  gcos\theta\]

\[ \Rightarrow  a_t  =  - \left( \frac{5}{7} \right)  gcos\theta\]

(c) At \[\theta = 0,\] from the free body diagram, we have

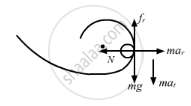
Normal force = \[N = m a_r\]

\[N = m \times \frac{10}{7}\frac{g\left( H - R - R\sin\theta \right)}{R}\]

\[= \left( \frac{70}{1000} \right) \times \left( \frac{10}{7} \right) \times 10  \left\{ \frac{0 . 6 - 0 . 1}{0 . 1} \right\}\]

\[=   5  N\]

At \[\theta = 0,\] from the free body diagram, we get

\[f_r  = mg - m a_t..........\left(f_r=\text{ Force of friction}\right)\]

\[\Rightarrow  f_r  = m\left( g - a_t \right)\]

\[= m\left( 10 - \frac{5}{7} \times 10 \right)\]

\[ = 0 . 07\left( 10 - \frac{5}{7} \times 10 \right)\]

\[= \frac{1}{100}  \left( 70 - 50 \right) = 0 . 2  N\]