Advertisements
Advertisements
प्रश्न
The given figure gives a speed-time graph of a particle in motion along a constant direction. Three equal intervals of time are shown. In which interval is the average acceleration greatest in magnitude? In which interval is the average speed greatest? Choosing the positive direction as the constant direction of motion, give the signs of v and a in the three intervals. What are the accelerations at the points A, B, C and D?

उत्तर
- The average acceleration's magnitude is determined by
= `"Change in speed"/"Time interval"`
The average acceleration over a brief period of time matches the v-t graph's slope during that specific interval. The v-t graph's slope is maximum during interval 2 when compared with intervals 1 and 3, therefore the greatest average acceleration in magnitude is observed in interval 2. - Interval 3 has the highest average speed since peak D reaches the maximum on the speed axis.
- v > 0 is positive throughout all three time intervals.
- The graph's slope is positive in intervals 1 and 3, which means a > 0; thus, acceleration is positive in these intervals. Conversely, the slope is negative in interval 2, indicating a negative acceleration. Hence, a > 0 signifies a positive acceleration in intervals 1 and 3, and a < 0 indicates a negative acceleration in interval 2.
- Since the slope is zero at points A, B, C, and D, the acceleration at these points is also zero.
APPEARS IN
संबंधित प्रश्न
Read the statement below carefully and state, with reason and example, if it is true or false:
A particle in one-dimensional motion with constant speed must have zero acceleration.
Read the statement below carefully and state, with reason and example, if it is true or false:
A particle in one-dimensional motion with positive value of acceleration must be speeding up.
Give example where the velocity is opposite in direction to the acceleration.
Give example where the velocity is perpendicular to the acceleration.
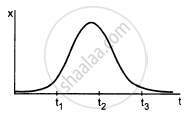
In figure shows the x coordinate of a particle as a function of time. Find the sings of vx and ax at t = t1, t = t2 and t = t3.
A stone is released from an elevator going up with an acceleration a. The acceleration of the stone after the release is
The displacement of particle is given by x = `2/lambda (1 - e^(-lambdat))`, the acceleration of particle at 2s when λ = 2s–1 will be (Take `l`n 0.018 = – 4)
A lift is coming from 8th floor and is just about to reach 4th floor. Taking ground floor as origin and positive direction upwards for all quantities, which one of the following is correct?
A graph of x versus t is shown in figure. Choose correct alternatives from below.
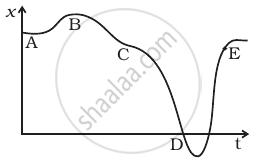
- The particle was released from rest at t = 0.
- At B, the acceleration a > 0.
- At C, the velocity and the acceleration vanish.
- Average velocity for the motion between A and D is positive.
- The speed at D exceeds that at E.
An object falling through a fluid is observed to have acceleration given by a = g – bv where g = gravitational acceleration and b is constant. After a long time of release, it is observed to fall with constant speed. What must be the value of constant speed?
