Advertisements
Advertisements
प्रश्न
The given figure shows the parallelograms ABCD and APQR.
Show that these parallelograms are equal in the area.
[ Join B and R ]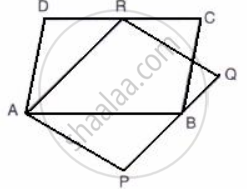
Advertisements
उत्तर
Join B and R and P and R.
We know that the area of the parallelogram is equal to twice the area of the triangle if the triangle and the parallelogram are on the same base and between the parallels.
Consider ABCD parallelogram:
Since the parallelogram ABCD and the triangle ABR lie on AB and between the parallels AB and DC, we have
Area(`square`ABCD ) = 2 x Area( ΔABR ) ....(1)
We know that the area of triangles with the same base and between the same parallel lines are equal.
Since the triangles ABR and APR lie on the same base AR and between the parallels AR and QP, we have,
Area ( ΔABR ) = Area ( ΔAPR ) ....(2)
From equations (1) and (2), we have,
Area(`square`ABCD) = 2 x Area( ΔAPR ) .....(3)
Also, the triangle APR and the parallelograms, AR and QR, lie on the same base AR and between the parallels, AR and QP,
Area( ΔAPR ) = `1/2` x Area(`square`ARQP ) ....(4)
Using (4) in equation (3), We have,
Area(`square`ABCD ) = 2 x `1/2 xx "Area"( square`ARQP )
Area( `square"ABCD" ) = "Area"( square` ARQP)
Hence Proved.
APPEARS IN
संबंधित प्रश्न
In the given figure, ABCD is a parallelogram; BC is produced to point X.
Prove that: area ( Δ ABX ) = area (`square`ACXD )
ABCD and BCFE are parallelograms. If area of triangle EBC = 480 cm2; AB = 30 cm and BC = 40 cm.
Calculate :
(i) Area of parallelogram ABCD;
(ii) Area of the parallelogram BCFE;
(iii) Length of altitude from A on CD;
(iv) Area of triangle ECF.
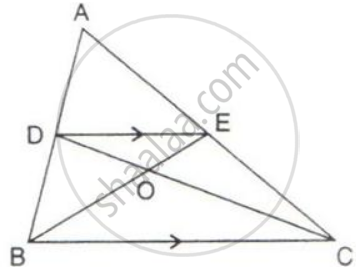
In the following figure, CE is drawn parallel to diagonals DB of the quadrilateral ABCD which meets AB produced at point E.
Prove that ΔADE and quadrilateral ABCD are equal in area.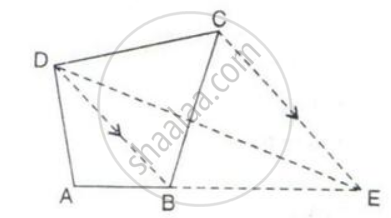
In the given figure, AP is parallel to BC, BP is parallel to CQ.
Prove that the area of triangles ABC and BQP are equal.
ABCD is a parallelogram a line through A cuts DC at point P and BC produced at Q. Prove that triangle BCP is equal in area to triangle DPQ.
ABCD is a parallelogram in which BC is produced to E such that CE = BC and AE intersects CD at F.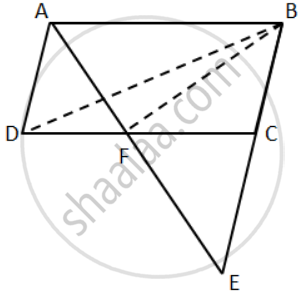
If ar.(∆DFB) = 30 cm2; find the area of parallelogram.
In a parallelogram ABCD, point P lies in DC such that DP: PC = 3:2. If the area of ΔDPB = 30 sq. cm.
find the area of the parallelogram ABCD.
ABCD is a parallelogram. P and Q are the mid-points of sides AB and AD respectively.
Prove that area of triangle APQ = `1/8` of the area of parallelogram ABCD.
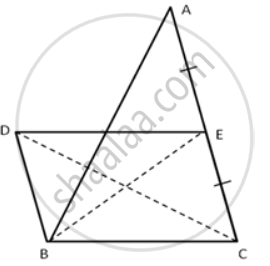
In parallelogram ABCD, E is a point in AB and DE meets diagonal AC at point F. If DF: FE = 5:3 and area of ΔADF is 60 cm2; find
(i) area of ΔADE.
(ii) if AE: EB = 4:5, find the area of ΔADB.
(iii) also, find the area of parallelogram ABCD.
In parallelogram ABCD, P is the mid-point of AB. CP and BD intersect each other at point O. If the area of ΔPOB = 40 cm2, and OP: OC = 1:2, find:
(i) Areas of ΔBOC and ΔPBC
(ii) Areas of ΔABC and parallelogram ABCD.
