Advertisements
Advertisements
प्रश्न
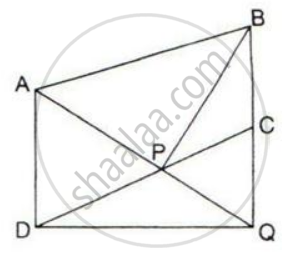
ABCD is a parallelogram a line through A cuts DC at point P and BC produced at Q. Prove that triangle BCP is equal in area to triangle DPQ.
उत्तर
ΔAPB and parallelogram ABCD are on the same base AB and between the same parallel lines AB and CD.
∴ Ar. ( ΔAPB ) = `1/2` Ar.( parallelogram ABCD ) ......(i)
ΔADQ and parallelogram ABCD are on the same base AD and between the same parallel lines AD and BQ.
∴ Ar.( ΔADQ ) = `1/2` Ar.( parallelogram ABCD ) ......(ii)
Adding equation (i) and (ii), we get
∴ Ar.( ΔAPB ) + Ar.( ΔADQ ) = Ar.(parallelogram ABCD)
Ar.( quad ADQB ) - Ar.(Δ BPQ ) = Ar.(parallelogram ABCD)
Ar.( quad ADQB) - Ar.( ΔBPQ ) = Ar.(quad ADQB ) -Ar.( ΔDCQ )
Ar. ( ΔBPQ ) = Ar. ( ΔDCQ )
Subtracting Ar.ΔPCQ from both sides, we get
Ar. ( ΔBPQ ) - Ar.(ΔPCQ ) = Ar. ( ΔDCQ ) - Ar. ( ΔPCQ)
Ar. ( ΔBCP ) = Ar. ( ΔDPQ )
Hence proved.
APPEARS IN
संबंधित प्रश्न
In the given figure, if the area of triangle ADE is 60 cm2, state, given reason, the area of :
(i) Parallelogram ABED;
(ii) Rectangle ABCF;
(iii) Triangle ABE.
In the given figure, ABCD is a parallelogram; BC is produced to point X.
Prove that: area ( Δ ABX ) = area (`square`ACXD )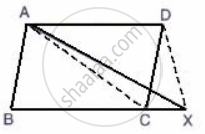
In the following, AC // PS // QR and PQ // DB // SR.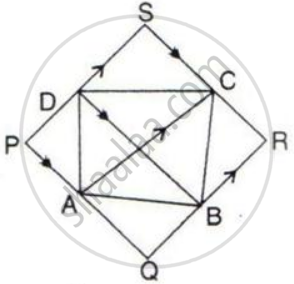
Prove that: Area of quadrilateral PQRS = 2 x Area of the quad. ABCD.
In the given figure, M and N are the mid-points of the sides DC and AB respectively of the parallelogram ABCD.
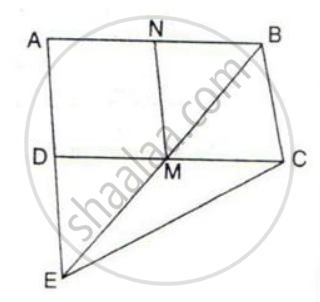
If the area of parallelogram ABCD is 48 cm2;
(i) State the area of the triangle BEC.
(ii) Name the parallelogram which is equal in area to the triangle BEC.
In the figure given alongside, squares ABDE and AFGC are drawn on the side AB and the hypotenuse AC of the right triangle ABC.
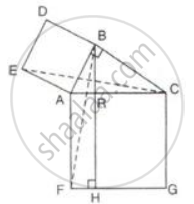
If BH is perpendicular to FG
prove that:
- ΔEAC ≅ ΔBAF
- Area of the square ABDE
- Area of the rectangle ARHF.
ABCD is a parallelogram. P and Q are the mid-points of sides AB and AD respectively.
Prove that area of triangle APQ = `1/8` of the area of parallelogram ABCD.
The given figure shows a parallelogram ABCD with area 324 sq. cm. P is a point in AB such that AP: PB = 1:2
Find The area of Δ APD.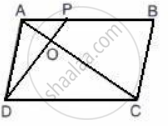
In ΔABC, E and F are mid-points of sides AB and AC respectively. If BF and CE intersect each other at point O,
prove that the ΔOBC and quadrilateral AEOF are equal in area.
In parallelogram ABCD, P is the mid-point of AB. CP and BD intersect each other at point O. If the area of ΔPOB = 40 cm2, and OP: OC = 1:2, find:
(i) Areas of ΔBOC and ΔPBC
(ii) Areas of ΔABC and parallelogram ABCD.
Show that:
The ratio of the areas of two triangles on the same base is equal to the ratio of their heights.
