Advertisements
Advertisements
प्रश्न
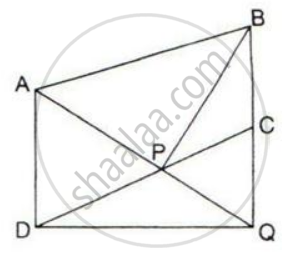
ABCD is a parallelogram a line through A cuts DC at point P and BC produced at Q. Prove that triangle BCP is equal in area to triangle DPQ.
उत्तर
ΔAPB and parallelogram ABCD are on the same base AB and between the same parallel lines AB and CD.
∴ Ar. ( ΔAPB ) = `1/2` Ar.( parallelogram ABCD ) ......(i)
ΔADQ and parallelogram ABCD are on the same base AD and between the same parallel lines AD and BQ.
∴ Ar.( ΔADQ ) = `1/2` Ar.( parallelogram ABCD ) ......(ii)
Adding equation (i) and (ii), we get
∴ Ar.( ΔAPB ) + Ar.( ΔADQ ) = Ar.(parallelogram ABCD)
Ar.( quad ADQB ) - Ar.(Δ BPQ ) = Ar.(parallelogram ABCD)
Ar.( quad ADQB) - Ar.( ΔBPQ ) = Ar.(quad ADQB ) -Ar.( ΔDCQ )
Ar. ( ΔBPQ ) = Ar. ( ΔDCQ )
Subtracting Ar.ΔPCQ from both sides, we get
Ar. ( ΔBPQ ) - Ar.(ΔPCQ ) = Ar. ( ΔDCQ ) - Ar. ( ΔPCQ)
Ar. ( ΔBCP ) = Ar. ( ΔDPQ )
Hence proved.
APPEARS IN
संबंधित प्रश्न
The given figure shows the parallelograms ABCD and APQR.
Show that these parallelograms are equal in the area.
[ Join B and R ]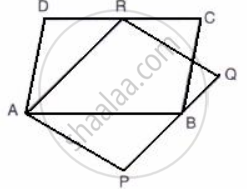
The given figure shows a rectangle ABDC and a parallelogram ABEF; drawn on opposite sides of AB.
Prove that:
(i) Quadrilateral CDEF is a parallelogram;
(ii) Area of the quad. CDEF
= Area of rect. ABDC + Area of // gm. ABEF.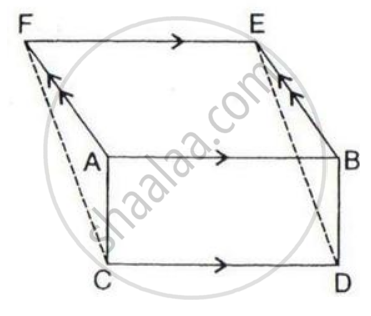
In the following, AC // PS // QR and PQ // DB // SR.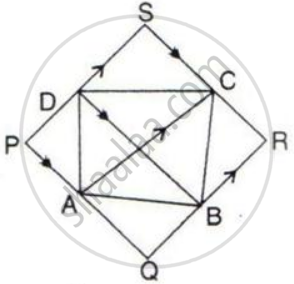
Prove that: Area of quadrilateral PQRS = 2 x Area of the quad. ABCD.
In the given figure, diagonals PR and QS of the parallelogram PQRS intersect at point O and LM is parallel to PS. Show that:
(i) 2 Area (POS) = Area (// gm PMLS)
(ii) Area (POS) + Area (QOR) = Area (// gm PQRS)
(iii) Area (POS) + Area (QOR) = Area (POQ) + Area (SOR).
In the given figure, M and N are the mid-points of the sides DC and AB respectively of the parallelogram ABCD.
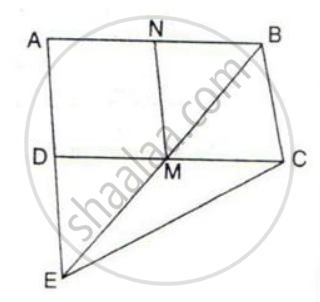
If the area of parallelogram ABCD is 48 cm2;
(i) State the area of the triangle BEC.
(ii) Name the parallelogram which is equal in area to the triangle BEC.
In the given figure, AP is parallel to BC, BP is parallel to CQ.
Prove that the area of triangles ABC and BQP are equal.
ABCD is a parallelogram. P and Q are the mid-points of sides AB and AD respectively.
Prove that area of triangle APQ = `1/8` of the area of parallelogram ABCD.
ABCD is a trapezium with AB parallel to DC. A line parallel to AC intersects AB at X and BC at Y.
Prove that the area of ∆ADX = area of ∆ACY.
In the following figure, BD is parallel to CA, E is mid-point of CA and BD = `1/2`CA
Prove that: ar. ( ΔABC ) = 2 x ar.( ΔDBC )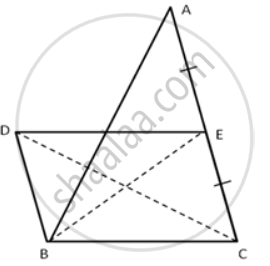
In ΔABC, E and F are mid-points of sides AB and AC respectively. If BF and CE intersect each other at point O,
prove that the ΔOBC and quadrilateral AEOF are equal in area.
