Advertisements
Advertisements
प्रश्न
ABCD is a parallelogram. P and Q are the mid-points of sides AB and AD respectively.
Prove that area of triangle APQ = `1/8` of the area of parallelogram ABCD.
उत्तर
We have to join PD and BD.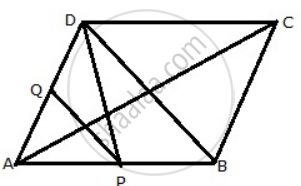
BD is the diagonal of the parallelogram ABCD. Therefore it divides the parallelogram into two equal parts.
∴ Area( ΔABD )= Area ( ΔDBC )
=`1/2` Area ( parallelogram ABCD) ...(i)
DP is the median of ΔABD. Therefore it will divide ΔABD into two triangles of equal areas.
∴ Area( ΔAPD )= Area ( ΔDPB )
= `1/2` Area ( ΔABD )
= `1/2 xx 1/2` Area (parallelogram ABCD) ...[from equation (i)]
= `1/4` Area (parallelogram ABCD) ...(ii)
In ΔAPD, Q is the mid-point of AD. Therefore PQ is the median.
∴ Area(ΔAPQ)= Area (ΔDPQ)
= `1/2` Area (ΔAPD)
= `1/2 xx 1/4` Area (parallelogram ABCD)...[from equation (ii)]
Area (ΔAPQ)= `1/8` Area (parallelogram ABCD),
hence proved
APPEARS IN
संबंधित प्रश्न
In the given figure, if the area of triangle ADE is 60 cm2, state, given reason, the area of :
(i) Parallelogram ABED;
(ii) Rectangle ABCF;
(iii) Triangle ABE.
The given figure shows the parallelograms ABCD and APQR.
Show that these parallelograms are equal in the area.
[ Join B and R ]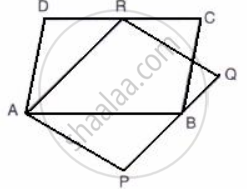
In the given figure, AD // BE // CF.
Prove that area (ΔAEC) = area (ΔDBF)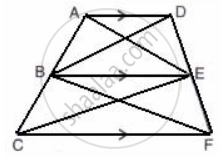
In the given figure, diagonals PR and QS of the parallelogram PQRS intersect at point O and LM is parallel to PS. Show that:
(i) 2 Area (POS) = Area (// gm PMLS)
(ii) Area (POS) + Area (QOR) = Area (// gm PQRS)
(iii) Area (POS) + Area (QOR) = Area (POQ) + Area (SOR).
In parallelogram ABCD, P is a point on side AB and Q is a point on side BC.
Prove that:
(i) ΔCPD and ΔAQD are equal in the area.
(ii) Area (ΔAQD) = Area (ΔAPD) + Area (ΔCPB)
ABCD is a parallelogram a line through A cuts DC at point P and BC produced at Q. Prove that triangle BCP is equal in area to triangle DPQ.
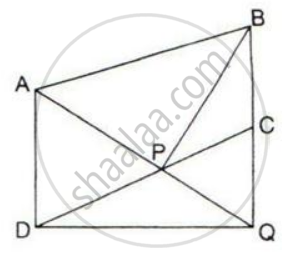
In a parallelogram ABCD, point P lies in DC such that DP: PC = 3:2. If the area of ΔDPB = 30 sq. cm.
find the area of the parallelogram ABCD.
E, F, G, and H are the midpoints of the sides of a parallelogram ABCD.
Show that the area of quadrilateral EFGH is half of the area of parallelogram ABCD.
In parallelogram ABCD, P is the mid-point of AB. CP and BD intersect each other at point O. If the area of ΔPOB = 40 cm2, and OP: OC = 1:2, find:
(i) Areas of ΔBOC and ΔPBC
(ii) Areas of ΔABC and parallelogram ABCD.
Show that:
The ratio of the areas of two triangles on the same base is equal to the ratio of their heights.
