Advertisements
Advertisements
प्रश्न
In parallelogram ABCD, P is the mid-point of AB. CP and BD intersect each other at point O. If the area of ΔPOB = 40 cm2, and OP: OC = 1:2, find:
(i) Areas of ΔBOC and ΔPBC
(ii) Areas of ΔABC and parallelogram ABCD.
उत्तर
(i) Joining AC we have the following figure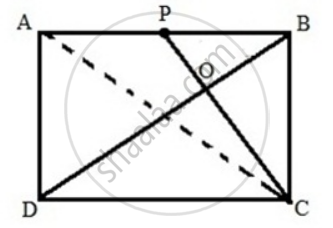
Consider the triangles ΔPOB and ΔCOD
∠POB = ∠DOC ...[ vertically opposite angles ]
∠OPB = ∠ODC ...[ AB and DC are parallel, CP and BD are the transversals, alternate interior angles are equal ]
Therefore, by Angle-Angle similarly criterion of congruence, ΔPOB ∼ ΔCOD
Since P is the mid-point AP = BP, and AB = CD, we have CD = 2 BP
Therefore, We have,
`"BP"/"CD" = "OP"/"OC"= "OB"/"OD" = 1/2`
⇒ OP : OC = 1: 2
(ii) Since from part ( i ), we have
`"BP"/"CD" = "OP"/"OC"= "OB"/"OD" = 1/2` ,
The ratio between the areas of two similar triangles is equal to the ratio between the square of the corresponding sides.
Here, ΔDOC and ΔPOB are similar triangles.
Thus, we have ,
`" Ar.( ΔDOC)"/"Ar.( ΔPOB )" ="DC"^2/"PB"^2"`
⇒ `"Ar.(ΔDOC )"/"Ar.( ΔPOB )" ="(2PB)"^2/"PB"^2"`
⇒ `"Ar.( ΔDOC )"/"Ar.( ΔPOB )" ="4PB"^2/"PB"^2"`
⇒ `"Ar.( ΔDOC )"/"Ar.( ΔPOB )"` = 4
⇒ Ar.( ΔDOC ) = 4Ar, ( ΔPOB )
= 4 x 40
= 160 cm2
Now consider Ar. ( ΔDBC ) = Ar. ( ΔDOC ) + Ar. (Δ BOC )
= 160 + 80
= 160 cm2
Two triangles are equal in the area if they are on equal bases and between the same parallels.
Therefore, Ar. ( ΔDBC ) = Ar. ( ΔABC ) = 240 cm2
The median divides the triangles into areas of two equal triangles.
Thus, CP is the median of the triangle ABC.
Hence, Ar. ( ΔABC ) = 2 Ar. ( ΔPBC )
Ar. ( ΔPBC ) = `"Ar.( ΔABC )"/2`
Ar. ( ΔPBC ) = 120 cm2
( iii ) From part (ii) we have,
Ar. ( ΔABC ) = 2Ar. ( PBC ) = 240 cm2
The area of a triangle is half the area of the parallelogram if both are on equal bases and between the same parallels.
Thus, Ar. ( ΔABC ) = `1/2` Ar. [ || gm ABCD ]
AR. [ || gm ABCD ] = 2 Ar. ( ΔABC )
AR. [ || gm ABCD ] = 2 x 240
AR. [ || gm ABCD ] = 480 cm2
APPEARS IN
संबंधित प्रश्न
The given figure shows a rectangle ABDC and a parallelogram ABEF; drawn on opposite sides of AB.
Prove that:
(i) Quadrilateral CDEF is a parallelogram;
(ii) Area of the quad. CDEF
= Area of rect. ABDC + Area of // gm. ABEF.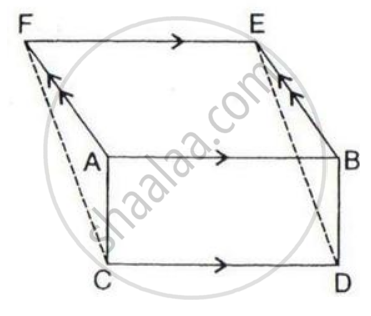
In the given figure, ABCD is a parallelogram; BC is produced to point X.
Prove that: area ( Δ ABX ) = area (`square`ACXD )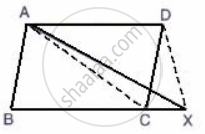
In the given figure, AD // BE // CF.
Prove that area (ΔAEC) = area (ΔDBF)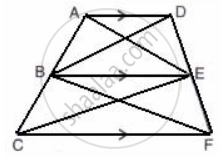
In the following, AC // PS // QR and PQ // DB // SR.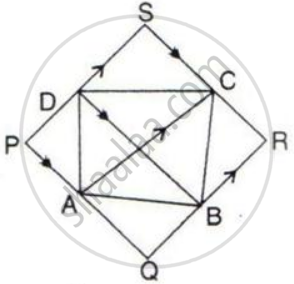
Prove that: Area of quadrilateral PQRS = 2 x Area of the quad. ABCD.
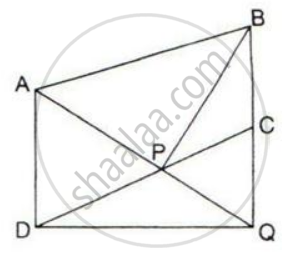
In the given figure, AP is parallel to BC, BP is parallel to CQ.
Prove that the area of triangles ABC and BQP are equal.
ABCD is a parallelogram a line through A cuts DC at point P and BC produced at Q. Prove that triangle BCP is equal in area to triangle DPQ.
Show that:
A diagonal divides a parallelogram into two triangles of equal area.
ABCD is a parallelogram in which BC is produced to E such that CE = BC and AE intersects CD at F.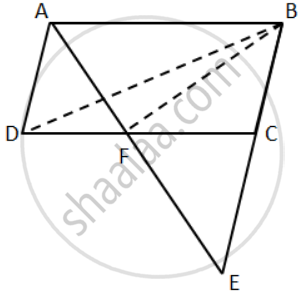
If ar.(∆DFB) = 30 cm2; find the area of parallelogram.
ABCD is a trapezium with AB parallel to DC. A line parallel to AC intersects AB at X and BC at Y.
Prove that the area of ∆ADX = area of ∆ACY.
In the following figure, BD is parallel to CA, E is mid-point of CA and BD = `1/2`CA
Prove that: ar. ( ΔABC ) = 2 x ar.( ΔDBC )