Advertisements
Advertisements
प्रश्न
In ΔABC, E and F are mid-points of sides AB and AC respectively. If BF and CE intersect each other at point O,
prove that the ΔOBC and quadrilateral AEOF are equal in area.
उत्तर
E and F are the midpoints of the sides AB and AC.
Consider the following figure.
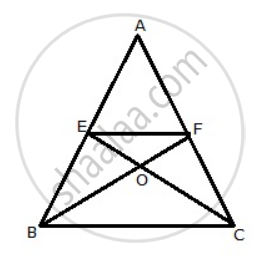
Therefore, by midpoint theorem, we have, EF || BC
Triangles BEF and CEF lie on the common base EF and between the parallels, EF and BC
Therefore, Ar.( ΔBEF ) = Ar.( ΔCOF )
⇒ Ar.( ΔBOE ) + Ar.( ΔEOF ) = Ar.( ΔEOF ) + Ar.( ΔCOF )
⇒ Ar.(ΔBOE ) = Ar.( ΔCOF )
Now BF and CE are the medians of the triangle ABC
Medians of the triangle divide it into two equal areas of triangles.
Thus, we have, Ar. (ΔABF) = Ar. (ΔCBF)
Subtracting Ar. ΔBOE on both the sides, we have
Ar. (ΔABF) - Ar. (ΔBOE) = Ar. (ΔCBF) - Ar. (ΔBOE)
Since, Ar. ( ΔBOE ) = Ar. ( ΔCOF ),
Ar. (ΔABF) - Ar. (ΔBOE) = Ar. (ΔCBF) - Ar. (ΔCOF)
Ar. ( quad. AEOF ) = Ar. ( ΔOBC ) , hence proved
APPEARS IN
संबंधित प्रश्न
In the given figure, if the area of triangle ADE is 60 cm2, state, given reason, the area of :
(i) Parallelogram ABED;
(ii) Rectangle ABCF;
(iii) Triangle ABE.
The given figure shows the parallelograms ABCD and APQR.
Show that these parallelograms are equal in the area.
[ Join B and R ]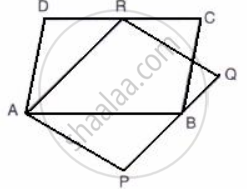
In the given figure, AD // BE // CF.
Prove that area (ΔAEC) = area (ΔDBF)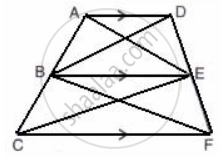
In the following, AC // PS // QR and PQ // DB // SR.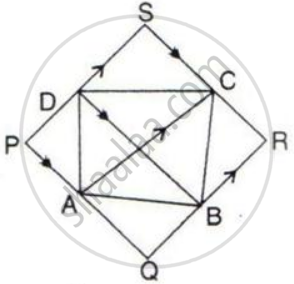
Prove that: Area of quadrilateral PQRS = 2 x Area of the quad. ABCD.
ABCD and BCFE are parallelograms. If area of triangle EBC = 480 cm2; AB = 30 cm and BC = 40 cm.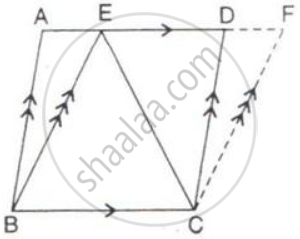
Calculate :
(i) Area of parallelogram ABCD;
(ii) Area of the parallelogram BCFE;
(iii) Length of altitude from A on CD;
(iv) Area of triangle ECF.
In the given figure, AP is parallel to BC, BP is parallel to CQ.
Prove that the area of triangles ABC and BQP are equal.
ABCD is a trapezium with AB parallel to DC. A line parallel to AC intersects AB at X and BC at Y.
Prove that the area of ∆ADX = area of ∆ACY.
E, F, G, and H are the midpoints of the sides of a parallelogram ABCD.
Show that the area of quadrilateral EFGH is half of the area of parallelogram ABCD.
In parallelogram ABCD, E is a point in AB and DE meets diagonal AC at point F. If DF: FE = 5:3 and area of ΔADF is 60 cm2; find
(i) area of ΔADE.
(ii) if AE: EB = 4:5, find the area of ΔADB.
(iii) also, find the area of parallelogram ABCD.
Show that:
The ratio of the areas of two triangles of the same height is equal to the ratio of their bases.
