Advertisements
Advertisements
प्रश्न
E, F, G, and H are the midpoints of the sides of a parallelogram ABCD.
Show that the area of quadrilateral EFGH is half of the area of parallelogram ABCD.
उत्तर
Join HF.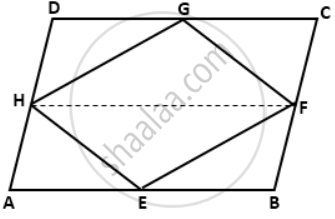
Since H and F are mid-points of AD and BC respectively,
∴ AH = `1/2 "AD and BF" = 1/2 "BC"`
Now, ABCD is a parallelogram.
⇒ AD = BC and AD ∥ BC
⇒ `1/2 "AD" = 1/2`BC and AD || BC
⇒ AH = BF and AH ∥ BF
⇒ ABFH is a parallelogram.
Since parallelogram FHAB and ΔFHE are on the same base FH and between the same parallels HF and AB,
A( ΔFHE ) = `1/2`A ( ||m FHAB ) .....(i)
Similarly,
A( ΔFHG ) = `1/2`A ( ||m FHDC ) .......(ii)
Adding (i) and (ii), We get,
A( ΔFHE ) + A( ΔFHG ) = `1/2 "A"( ||^m "FHAB" ) + 1/2`A ( ||m FHDC )
⇒ A( EFGH ) = `1/2`[ A ( ||m FHAB ) + A ( ||m FHDC ) ]
⇒ A( EFGH ) = `1/2`A( ||m ABCD )
APPEARS IN
संबंधित प्रश्न
In the given figure, D is mid-point of side AB of ΔABC and BDEC is a parallelogram.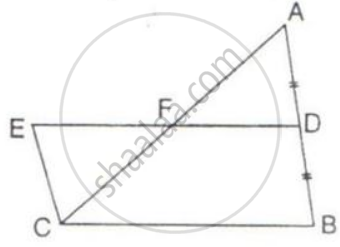
Prove that: Area of ABC = Area of // gm BDEC.
ABCD and BCFE are parallelograms. If area of triangle EBC = 480 cm2; AB = 30 cm and BC = 40 cm.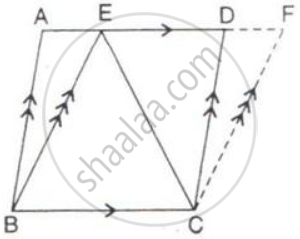
Calculate :
(i) Area of parallelogram ABCD;
(ii) Area of the parallelogram BCFE;
(iii) Length of altitude from A on CD;
(iv) Area of triangle ECF.
In the following figure, DE is parallel to BC.
Show that:
(i) Area ( ΔADC ) = Area( ΔAEB ).
(ii) Area ( ΔBOD ) = Area( ΔCOE ).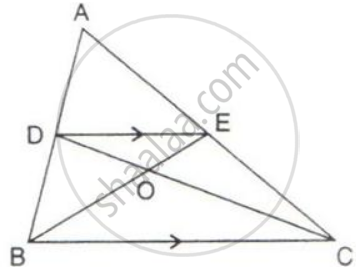
In the following figure, CE is drawn parallel to diagonals DB of the quadrilateral ABCD which meets AB produced at point E.
Prove that ΔADE and quadrilateral ABCD are equal in area.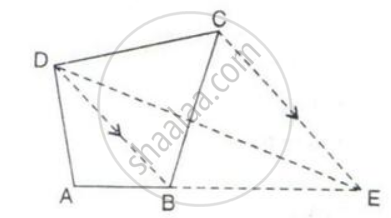
In the figure given alongside, squares ABDE and AFGC are drawn on the side AB and the hypotenuse AC of the right triangle ABC.
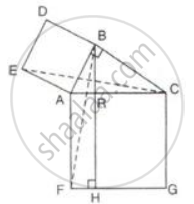
If BH is perpendicular to FG
prove that:
- ΔEAC ≅ ΔBAF
- Area of the square ABDE
- Area of the rectangle ARHF.
ABCD is a parallelogram a line through A cuts DC at point P and BC produced at Q. Prove that triangle BCP is equal in area to triangle DPQ.
ABCD is a parallelogram in which BC is produced to E such that CE = BC and AE intersects CD at F.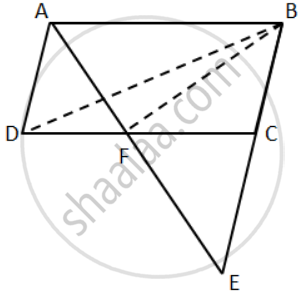
If ar.(∆DFB) = 30 cm2; find the area of parallelogram.
In a parallelogram ABCD, point P lies in DC such that DP: PC = 3:2. If the area of ΔDPB = 30 sq. cm.
find the area of the parallelogram ABCD.
In parallelogram ABCD, E is a point in AB and DE meets diagonal AC at point F. If DF: FE = 5:3 and area of ΔADF is 60 cm2; find
(i) area of ΔADE.
(ii) if AE: EB = 4:5, find the area of ΔADB.
(iii) also, find the area of parallelogram ABCD.
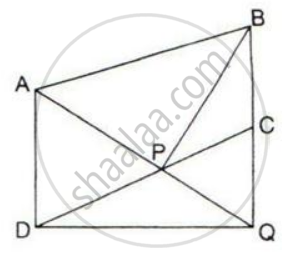
In parallelogram ABCD, P is the mid-point of AB. CP and BD intersect each other at point O. If the area of ΔPOB = 40 cm2, and OP: OC = 1:2, find:
(i) Areas of ΔBOC and ΔPBC
(ii) Areas of ΔABC and parallelogram ABCD.
