Advertisements
Advertisements
Question
E, F, G, and H are the midpoints of the sides of a parallelogram ABCD.
Show that the area of quadrilateral EFGH is half of the area of parallelogram ABCD.
Solution
Join HF.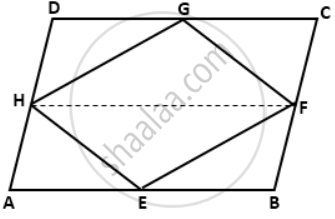
Since H and F are mid-points of AD and BC respectively,
∴ AH = `1/2 "AD and BF" = 1/2 "BC"`
Now, ABCD is a parallelogram.
⇒ AD = BC and AD ∥ BC
⇒ `1/2 "AD" = 1/2`BC and AD || BC
⇒ AH = BF and AH ∥ BF
⇒ ABFH is a parallelogram.
Since parallelogram FHAB and ΔFHE are on the same base FH and between the same parallels HF and AB,
A( ΔFHE ) = `1/2`A ( ||m FHAB ) .....(i)
Similarly,
A( ΔFHG ) = `1/2`A ( ||m FHDC ) .......(ii)
Adding (i) and (ii), We get,
A( ΔFHE ) + A( ΔFHG ) = `1/2 "A"( ||^m "FHAB" ) + 1/2`A ( ||m FHDC )
⇒ A( EFGH ) = `1/2`[ A ( ||m FHAB ) + A ( ||m FHDC ) ]
⇒ A( EFGH ) = `1/2`A( ||m ABCD )
APPEARS IN
RELATED QUESTIONS
In the given figure, if the area of triangle ADE is 60 cm2, state, given reason, the area of :
(i) Parallelogram ABED;
(ii) Rectangle ABCF;
(iii) Triangle ABE.
The given figure shows the parallelograms ABCD and APQR.
Show that these parallelograms are equal in the area.
[ Join B and R ]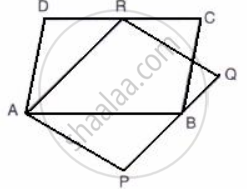
In the given figure, ABCD is a parallelogram; BC is produced to point X.
Prove that: area ( Δ ABX ) = area (`square`ACXD )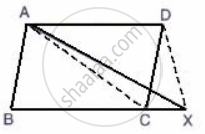
In the given figure, AD // BE // CF.
Prove that area (ΔAEC) = area (ΔDBF)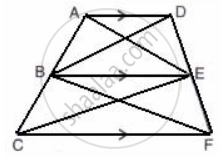
ABCD is a trapezium with AB // DC. A line parallel to AC intersects AB at point M and BC at point N.
Prove that: area of Δ ADM = area of Δ ACN.
In the given figure, M and N are the mid-points of the sides DC and AB respectively of the parallelogram ABCD.
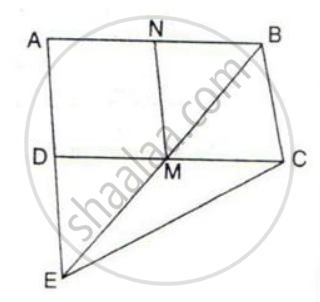
If the area of parallelogram ABCD is 48 cm2;
(i) State the area of the triangle BEC.
(ii) Name the parallelogram which is equal in area to the triangle BEC.
In the following figure, CE is drawn parallel to diagonals DB of the quadrilateral ABCD which meets AB produced at point E.
Prove that ΔADE and quadrilateral ABCD are equal in area.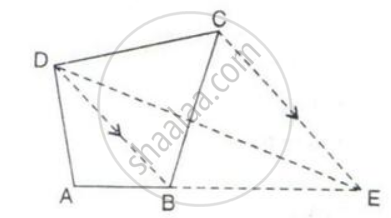
ABCD is a parallelogram in which BC is produced to E such that CE = BC and AE intersects CD at F.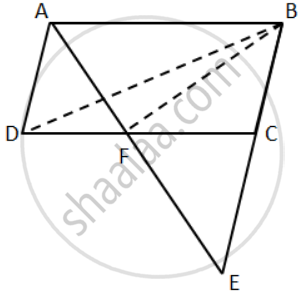
If ar.(∆DFB) = 30 cm2; find the area of parallelogram.
The given figure shows a parallelogram ABCD with area 324 sq. cm. P is a point in AB such that AP: PB = 1:2
Find The area of Δ APD.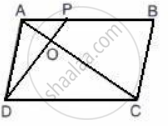
Show that:
The ratio of the areas of two triangles on the same base is equal to the ratio of their heights.
