Advertisements
Advertisements
Question
ABCD is a parallelogram in which BC is produced to E such that CE = BC and AE intersects CD at F.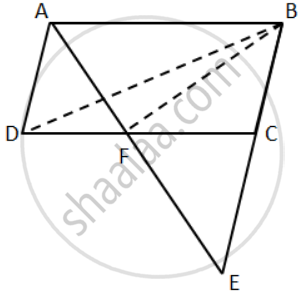
If ar.(∆DFB) = 30 cm2; find the area of parallelogram.
Solution

BC = CE .....( given )
Also, in parallelogram ABCD, BC = AD
⇒ AD = CE
Now, in ΔADF and ΔECF, We have
AD = CE
∠ADF = ∠ECF .....( Alternate angles )
∠DAF = ∠CEF ......( Alternate angles )
∴ ΔADF ≅ ΔECF ......( ASA Criterion )
⇒ Area( ΔADF ) = Area( ΔECF ) ....(1)
Also, in ΔFBE, FC is the median ....( Since BC = CE )
⇒ Area( ΔBCF ) = Area( ΔECF ) .....(2)
From (1) and (2)
Area( ΔADF ) = Area( ΔBCF ) ......(3)
Again, ΔADF and ΔBDF are on the base DF and between parallels DF and AB.
⇒ Area( ΔBDF ) = Area( ΔADF ) ........(4)
From (3) and (4),
Area( ΔBDF ) = Area( ΔBCF ) = 30 cm2
Area( ΔBCD ) = Area( ΔBDF ) + Area( ΔBCF ) = 30 + 30 = 60 cm2
Hence, Area of parallelogram ABCD = 2 x Area( ΔBCD ) = 2 x 60 = 120cm2.
APPEARS IN
RELATED QUESTIONS
The given figure shows a rectangle ABDC and a parallelogram ABEF; drawn on opposite sides of AB.
Prove that:
(i) Quadrilateral CDEF is a parallelogram;
(ii) Area of the quad. CDEF
= Area of rect. ABDC + Area of // gm. ABEF.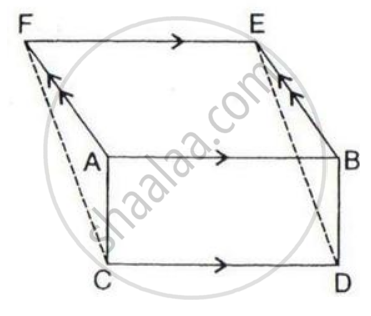
In the given figure, ABCD is a parallelogram; BC is produced to point X.
Prove that: area ( Δ ABX ) = area (`square`ACXD )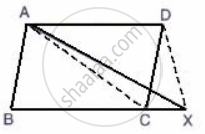
In the given figure, AD // BE // CF.
Prove that area (ΔAEC) = area (ΔDBF)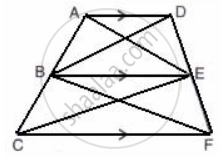
ABCD is a trapezium with AB // DC. A line parallel to AC intersects AB at point M and BC at point N.
Prove that: area of Δ ADM = area of Δ ACN.
In parallelogram ABCD, P is a point on side AB and Q is a point on side BC.
Prove that:
(i) ΔCPD and ΔAQD are equal in the area.
(ii) Area (ΔAQD) = Area (ΔAPD) + Area (ΔCPB)
In the following figure, CE is drawn parallel to diagonals DB of the quadrilateral ABCD which meets AB produced at point E.
Prove that ΔADE and quadrilateral ABCD are equal in area.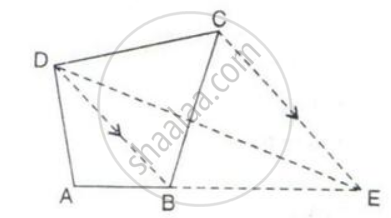
In the figure given alongside, squares ABDE and AFGC are drawn on the side AB and the hypotenuse AC of the right triangle ABC.
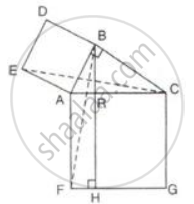
If BH is perpendicular to FG
prove that:
- ΔEAC ≅ ΔBAF
- Area of the square ABDE
- Area of the rectangle ARHF.
Show that:
A diagonal divides a parallelogram into two triangles of equal area.
ABCD is a parallelogram. P and Q are the mid-points of sides AB and AD respectively.
Prove that area of triangle APQ = `1/8` of the area of parallelogram ABCD.
In the given figure, the diagonals AC and BD intersect at point O. If OB = OD and AB//DC,
show that:
(i) Area (Δ DOC) = Area (Δ AOB).
(ii) Area (Δ DCB) = Area (Δ ACB).
(iii) ABCD is a parallelogram.

