Advertisements
Advertisements
प्रश्न
The length of a side of a square field is 4 m. what will be the altitude of the rhombus, if the area of the rhombus is equal to the square field and one of its diagonal is 2 m?
उत्तर
Length of the square field = 4 m
∴ A {rea of the square field = 4 x 4 = 16 m}2
Given: Area of the rhombus = Area of the square field
Length of one diagonal of the rhombus = 2 m
∴ Side of the rhombus \[=\frac{1}{2}\sqrt{d_1^2 + d_2^2}\]
And, area of the rhombus \[=\frac{1}{2} \times ( d_1 \times d_2 )\]
∴ Area:
\[16 = \frac{1}{2}(2 \times d_2 )\]
\[ d_2 =16 m\]
Now, we need to find the length of the side of the rhombus.
∴ Side of the rhombus \[=\frac{1}{2}\sqrt{2^2 + {16}^2}=\frac{1}{2}\sqrt{260}=\frac{1}{2}\sqrt{4 \times 65}=\frac{1}{2}\times2\sqrt{65}=\sqrt{65}m\]
Also, we know: Area of the rhombus = Side X Altitude
\[ \therefore 16=\sqrt{65}\times \] Altitude
Altitude \[=\frac{16}{\sqrt{65}}m\]
APPEARS IN
संबंधित प्रश्न
The diagonals of a rhombus are 7.5 cm and 12 cm. Find its area.
Find the area of a rhombus whose side is 5 cm and whose altitude is 4.8 cm. If one of its diagonals is 8 cm long, find the length of the other diagonal.
The floor of a building consists of 3000 tiles, which are rhombus shaped, and each of its diagonals are 45 cm and 30 cm in length. Find the total cost of polishing the floor if the cost per m2 is ₹ 4.
Find the area of the field in the form of a rhombus, if the length of each side be 14 cm and the altitude be 16 cm.
The area of a rhombus is 84 m2. If its perimeter is 40 m, then find its altitude.
A garden is in the form of a rhombus whose side is 30 metres and the corresponding altitude is 16 m. Find the cost of levelling the garden at the rate of Rs 2 per m2.
A field in the form of a rhombus has each side of length 64 m and altitude 16 m. What is the side of a square field which has the same area as that of a rhombus?
Find the area of the following polygon, if AL = 10 cm, AM = 20 cm, AN = 50 cm, AO = 60 cm and AD = 90 cm.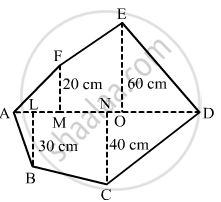
Find the area of the following regular hexagon.
